Advertisements
Advertisements
प्रश्न
50 circular plates each of diameter 14cm and thickness 0.5cm are placed one above other to form a right circular cylinder. Find its total surface area?
उत्तर
Given that 50 circular plates each with diameter = 14cm
Radius of circular plates (r) = 7cm
Thickness of plates= 0.5
Since these plates are placed one above other so total thickness of plates = 0. 5 x 50
= 25cm.
Total surface area of acylinder = 2πrh + 2πr2
= 2πrh + 2πr2
= 2πr (h+r)
= 2 x 22/7 x 7(25+7)
T.S.A = 1408cm2
∴Total surface area of circular plates is 1408cm2
APPEARS IN
संबंधित प्रश्न
A petrol tank is a cylinder of base diameter 21 cm and length 18 cm fitted with conical ends each of axis length 9 cm. Determine the capacity of the tank.
The adjoining figure represents a solid consisting of a cylinder surmounted by a cone at one end and a hemisphere at the other. Find the volume of the solid.

A solid right circular cone of height 60 cm and radius 30 cm is dropped in a right circular cylinder full of water, of height 180 cm and radius 60 cm. Find the volume of water left in the cylinder, in cubic metres.
The volume of a wall, 5 times as high as it is broad and 8 times as long as it is high, is 128 m3. The breadth of the wall is
The heights of two circular cylinders of equal volume are in the ratio 1 : 2. The ratio of their radii is
The radius of a solid right circular cylinder decreases by 20% and its height increases by 10%. Find the percentage change in its : volume
The radius of a solid right circular cylinder decreases by 20% and its height increases by 10%. Find the percentage change in its : curved surface area.
If the radius of base of a right circular cylinder is halved, keeping the height same, the ratio of the volume of the reduced cylinder to that of the original cylinder is ______.
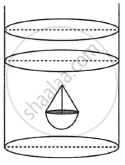
Two solid cones A and B are placed in a cylinderical tube as shown in the figure. The ratio of their capacities are 2:1. Find the heights and capacities of cones. Also, find the volume of the remaining portion of the cylinder
A solid is in the shape of a hemisphere of radius 7 cm, surmounted by a cone of height 4 cm. The solid is immersed completely in a cylindrical container filled with water to a certain height. If the radius of the cylinder is 14 cm, find the rise in the water level.