Advertisements
Advertisements
प्रश्न
A bus stop is barricated from the remaining part of the road, by using 50 hollow cones made of recycled card-board. Each cone has a base diameter of 40 cm and height 1 m. If the outer side of each of the cones is to be painted and the cost of painting is Rs. 12 per m2, what will be the cost of painting all these cones. (Use 𝜋 = 3.14 and √1.04 = 1.02)
उत्तर
Radius of cone`(r)=40/2=20m=0.2m`
Height of cone=1m
Slant height of cone(l)`=sqrt(h^2+r^2)`
=`sqrt(1^2+(0.2)^2m)`
=`sqrt(1.04m=1.02m)`
Curved surface area of each one
=`pirl=(3.14xx0.2xx1.02)m^2`
=` 0.64056m^2`
CSA of 50 such cone`=50xx0.64056m^2=32.028m^2`
Cost of painting` 1m^2` area=Rs.12.
Cost of painting` 32.028m^2 area=Rs(32.028xx12)`
= Rs.384.326PS
Thus, it will cost Rs. 38434 (Approx) in painting the so hollow cones.
APPEARS IN
संबंधित प्रश्न
A cone and a hemisphere have the same base and the same height. Find the ratio between their volumes.
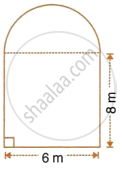
The cross-section of a railway tunnel is a rectangle 6 m broad and 8 m high surmounted by a semi-circle as shown in the figure. The tunnel is 35 m long. Find the cost of plastering the internal surface of the tunnel (excluding the floor) at the rate of Rs. 2.25 per m2.
The curved surface area of a cone is 2200 sq.cm and its slant height is 50 cm. Find the total surface area of cone. `(π = 22/7)`
Find the radius of the circular base of the cone , if its volume is 154 cm3 and the perpendicular height is 12 cm
Find the curved surface area of a cone whose height is 8 cm and base diameter is 12 cm .
The heights of two cones are in the ratio 1:3 and their base radii are in the ratio 3:1. Find the ratio of their volumes.
The radius and height of a cylinder, a cone and a sphere are same. Calculate the ratio of their volumes.
The volume of a conical tent is 1232 m3 and the area of the base floor is 154 m2. Calculate the: length of the canvas required to cover this conical tent if its width is 2 m.
The circumference of the base of a 10 m high conical tent is 44 metres. Calculate the length of canvas used in making the tent if the width of the canvas is 2m. (Take π = 22/7)
A vessel in the form of an inverted cone is filled with water to the brim: Its height is 20 cm and the diameter is 16.8 cm. Two equal solid cones are dropped in it so that they are fully submerged. As a result, one-third of the water in the original cone overflows. What is the volume of each of the solid cones submerged?
