Question

A cube of mass 'M' and side 'L' is fixed on the horizontal surface. The modulus of rigidity of the material of the cube is 'η'. A force is applied perpendicular to one of the side faces. When the force is removed, the cube executes small oscillations. The time period ______

विकल्प

• `2pisqrt(M/(ηL))` 

• `2pisqrt(η/(ML))`

• `M/(2pietaL)`

• `(2pietaL)/M`

Answer 1

A cube of mass 'M' and side 'L' is fixed on the horizontal surface. The modulus of rigidity of the material of the cube is 'η'. A force is applied perpendicular to one of the side faces. When the force is removed, the cube executes small oscillations. The time period `underline(2pisqrt(M/(ηL)))`.

Explanation:

 

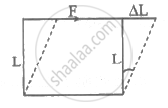
`eta = F/L^2 . L/(DeltaL)`

= `F/(LDeltaL)`

∴ F = ηLΔL

F = kΔL where k = ηL

∴ `T = 2pisqrt(M/k) = 2pisqrt(M/(etaL))`