Advertisements
Advertisements
प्रश्न
A dielectric slab of thickness 't’ is kept between the plates of a parallel plate capacitor with plate separation 'd' (t < d). Derive the expression for the capacitance of the capacitor.
उत्तर
P and Q are two parallel plates. The distance between them is d.
A dielectric material of thickness t(t < d) and dielectric constant K is inserted in between the plates.
In the remaining space (d - t) there is air.

The electric field in the dielectric-filled portion = `E_1 = sigma/(epsi_0K)`
The electric field in the air-filled portion = `E_2 = sigma/(epsi_0)`
The potential difference between the plates = V
V = E2 × (d - t) + E1 × t
Or, V = `sigma/(2epsi_0) xx (d - t) + sigma/(epsi_0K) xx t`
Or, V = `q/(Aepsi_0) xx [(d - t) + t/K]`
Now, capacitance = C = `q/V`
Or, C = `(epsi_0A)/((d - t) + t/K)`
APPEARS IN
संबंधित प्रश्न
Two parallel-plate capacitors X and Y have the same area of plates and same separation between them. X has air between the plates, while Y contains a dielectric medium of εr = 4.
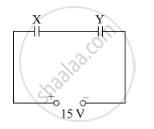
(i) Calculate capacitance of each capacitor if the equivalent capacitance of the combination is 4 μF.
(ii) Calculate the potential difference between the plates X and Y.
(iii) Estimate the ratio of electrostatic energies stored in X and Y.
A parallel plate capacitor with air between the plates has a capacitance of 8 pF (1pF = 10−12 F). What will be the capacitance if the distance between the plates is reduced by half, and the space between them is filled with a substance of dielectric constant 6?
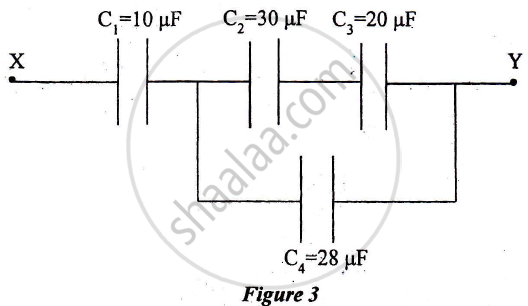
Four capacitors, C1, C2, C3 andC4 are connected as shown in Figure 3 below. Calculate equivalent capacitance of the circuit between points X . and Y.
A dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
Two parallel uniformly charged infinite plane sheets, '1' and '2', have charge densities + `sigma and -2sigma `respectively. Give the magnitude and direction of the net electric field at a point.
(i) in between the two sheets and
(ii) outside near the sheet '1'.
Two metal plates are separated by 2 cm. The potentials of the plates are -10 V and + 30 V. The electric field between the two plates is ______.
A parallel plate capacitor with air between the plates has a capacitance of 8 pF. Calculate the capacitance if the distance between the plates is reduced by half and the space between them is filled with a substance of dielectric constant. (εr = 6)
In a capacitor of capacitance 20 µF the distance between the plates is 5 mm. If a dielectric slab of width 2 mm and dielectric constant 3 is inserted between the plates, then the new capacitor will be ______.
Two infinitely long parallel conducting plates having surface charge densities +σ cand -σ respectively are separated by a small distance. The medium between the plates is a vacuum. If ε0 is the dielectric permittivity of the vacuum, then the electric field in the region between the plates is ______.
A dielectric slab of dielectric constant K and thickness t is introduced between the two plates of a capacitor of plate-separation d (>t) and common area A. The capacitance of this system is given as:
`C = (∈_o"A")/((d-t)+(t/k))`
How does the capacitance C modify in each of the following cases?
- The dielectric slab covers half the distance of separation between the two plates.
- The whole space between the plates is filled with the dielectric.
