Advertisements
Advertisements
प्रश्न
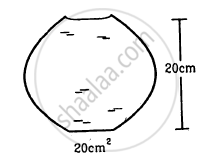
A glass full of water has a bottom of area 20 cm2, top of area 20 cm2, height 20 cm and volume half a litre.
(a) Find the force exerted by the water on the bottom.
(b) Considering the equilibrium of the water, find the resultant force exerted by the sides of the glass on the water. Atmospheric pressure = 1.0 × 105 N/m2. Density of water 1000 kg/m3 and g = 10 m/s2. Take all numbers
to be exact.
Advertisements
उत्तर
Given:
Atmospheric pressure, pa = 1.0 ×105N/m2
Density of water, ρw =103kg/m3
Acceleration due to gravity, g =10m/s2
Volume of water , V = 500 mL ≈ 500g ≈ 0.5 kg
Area of the top of the glass, A = 20 m2
Height of the glass, h = 20 cm
(a) Force exerted on the bottom of the glass = Atmospheric force + Force due to cylindrical water column or glass
=pa × A + A × h × ρw × g
=A(ρa + hρwg)
=20×10-4(105+20 ×10-2 ×103 ×10)
=204 N
(b) Let Fs be the force exerted by the sides of the glass. Now, from the free body diagram of water inside the glass, we can find out the resultant force exerted by the sides of the glass.
Thus, we have:
Pa × A + mg = A × h × ρw × g + Fs + Pa × A
⇒ mg = A × h × ρw × g + Fs
⇒ 0.5 × 1 = 20 × 10-4 × 20 × 10-2 × 10-3 × 10 + Fs
⇒ Fs = 5 - 4 = 1N (upward)
APPEARS IN
संबंधित प्रश्न
A U-tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms are in level with 10.0 cm of water in one arm and 12.5 cm of spirit in the other. What is the specific gravity of spirit?
Does it matter if one uses gauge instead of absolute pressures in applying Bernoulli’s equation? Explain.
A one meter long glass tube is open at both ends. One end of the tube is dipped into a mercury cup, the tube is kept vertical and the air is pumped out of the tube by connecting the upper end to a suction pump. Can mercury be pulled up into the pump by this process?
A satellite revolves round the earth. Air pressure inside the satellite is maintained at 76 cm of mercury. What will be the height of mercury column in a barometer tube 1 m long placed in the satellite?
The three vessels shown in the following figure have same base area. Equal volumes of a liquid are poured in the three vessels. The force on the base will be
Equal mass of three liquids are kept in three identical cylindrical vessels A, B and C. The densities are ρA, ρB, ρC with ρA < ρB < ρC. The force on the base will be
Shows in the following figure a siphon. The liquid shown is water. The pressure difference PB − PAbetween the points A and B is
Suppose the pressure at the surface of mercury in a barometer tube is P1 and the pressure at the surface of mercury in the cup is P2.
A barometer kept in an elevator accelerating upward reads 76 cm. The air pressure in the elevator is
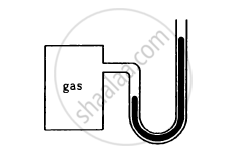
The heights of mercury surfaces in the two arms of the manometer shown in figure are 2 cm and 8 cm.
Atmospheric pressure = 1.01 × 105 N−2. Find (a) the pressure of the gas in the cylinder and (b) the pressure of mercury at the bottom of the U tube.
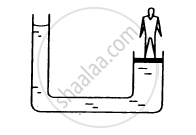
The area of cross section of the wider tube shown in figure is 900 cm2. If the boy standing on the piston weighs 45 kg, find the difference in the levels of water in the two tubes.
A closed vessel is half filled with water. There is a hole near the top of the vessel and air is pumped out from this hole.
(a) The water level will rise up in the vessel.
(b) The pressure at the surface of the water will decrease
(c) The force by the water on the bottom of the vessel will decrease
(d) The density of the liquid will decrease
Water is filled in a rectangular tank of size 3 m × 2 m × 1 m. (a) Find the total force exerted by the water on the bottom surface on the tank. (b) Consider a vertical side of area 2 m × 1 m. Take a horizontal strip of width δx metre in this side, situated at a depth of x metre from the surface of water. Find the force by the water on this strip. (c) Find the torque of the force calculate in part (b) about the bottom edge of this side.
(d) Find the total force by the water on this side.
(e) Find the total torque by the water on the side about the bottom edge. Neglect the atmospheric pressure and take g = 10 ms−2.
Pressure decreases as one ascends the atmosphere. If the density of air is ρ, what is the change in pressure dp over a differential height dh?
Considering the pressure p to be proportional to the density, find the pressure p at a height h if the pressure on the surface of the earth is p0.
A glass capillary sealed at the upper end is of length 0.11 m and internal diameter 2 × 10-5 m. This tube is immersed vertically into a liquid of surface tension 5.06 × 10-2 N/m. When the length x × 10-2 m of the tube is immersed in liquid then the liquid level inside and outside the capillary tube becomes the same, then the value of x is ______ m. (Assume atmospheric pressure is 1.01 × 105 `"N"/"m"^2`)
