Advertisements
Advertisements
प्रश्न
A ray of green light enters a liquid from air, as shown in Fig. The angle 1 is 45° and angle 2 is 30°.
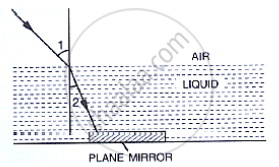
- Find the refractive index of the liquid.
- Show in the diagram the path of the ray after it strikes the mirror and re-enters in air. Mark in the diagram the angles where ever necessary.
- Redraw the diagram if plane mirror becomes normal to the refracted ray inside the liquid. State the principal used.
उत्तर
- Refractive index of the liquid =
`a ^µ i = "sin i" /"sin r"`
`a^ µ i= (sin45°)/(sin 30°)=(1//sqrt2)/(1//2)=sqrt2=1.414` - Path is shown
∠i = ∠45°, sin 45° = 0.7
∠r = 30° and sin 30° = 0.5
The path of the beam after it hits the mirror and re-enters the air is depicted in the labelled diagram below:
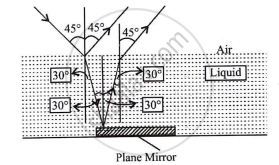
- The reversibility of the light path principle is the one that is applied.
APPEARS IN
संबंधित प्रश्न
A ray of light from air suffers partial reflection and refraction at the boundary of water. In Fig. 4.16, which of the ray A, B, C, D and E is the correct
(i) refracted ray
(ii) partially reflected ray?

A ray of light is moving from a rarer medium to a denser medium and strikes a plane mirror placed at 90° to the direction of the ray as shown in the diagram.

(i) Copy the diagram and mark arrows to show the path of the ray of light after it is reflected from the mirror.
(ii) Name the principle you have used to mark the arrows to show the direction of the ray.
