Question

A resistance and a capacitor connected in series across a 250V supply draws 5A at 50Hz. When frequency is increased to 60Hz, it draws 5.8A. Find the values of R & C. Also find active power and power factor in both cases.

Answer 1

𝐼₁=5𝐴  V = 250V   𝑓₁=50𝐻𝑧   𝑓₂=60𝐻𝑧   𝐼₂=5.8𝐴
(1) Values of R and C.

For 𝑓₁=50𝐻𝑧   𝑍₁=`V/I_1=250/5`= 50Ω

`Z_1^2=(R^2+(1/(2pif_1c))^2)=(R^2+(1/(100piC))^2)`

`R^2+(1/(100piC))^2=2500`…………….(1)

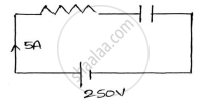
For 𝑓₁=60𝐻𝑧   `Z_2=V/I_2=250/5.8` = 43.1Ω

`Z_2^2=(R^2+1/(2pif_1c))=(R^2+(1/(100piC))^2)`

`R^2+(1/(120piC))^2`=1857.9Ω …………….(2)

From (1) and (2) we get,

R = 19.96Ω C = 69.4𝝁𝑭

(2) Power draw in the both cases.
𝑃₁= 𝐼₁²𝑅=5²×19.96 = 499w    𝑃₂= 𝐼₂²𝑅=5.8²×19.96 = 671.45w
𝑷_𝟏=𝟒𝟗𝟗𝒘 and 𝑷_𝟐= 671.45w

(3) Power factor in both cases:-
P₁=VI₁cosφ₁
499=250×5×cos𝜑₁ => cos𝜑₁=0.3992
𝝋_𝟏=𝟔𝟔.𝟒𝟕𝟏°
P₂=VI₂cosφ₂
671.45=250×5.8×cos𝜑₂ => cos𝜑₂=0.463
𝝋_𝟏=𝟔𝟐.𝟒𝟏𝟒𝟔°