Advertisements
Advertisements
प्रश्न
A rod AD of length 40 cm is suspended from point D as shown in figure. If it has a weight of 25 N and also supports a load of 40N,find the tension in the cable using the method of virtual work.Take AC=30 cm.
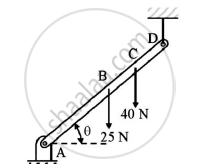
Given : Length of rod AD=40cm=0.4m
AC=0.3m
W=25N
Load on rod AD=40N
To find : Tension in the cable
उत्तर
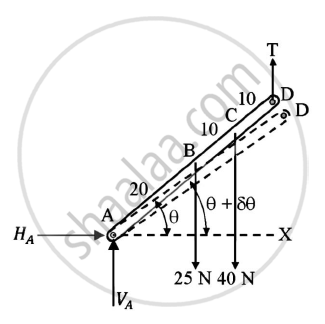
Assume rod AD have a small virtual angular displacement 𝛿θ in the clockwise direction T is the tension in the cable
Assume A be the origin and AX be the X-axis
Reaction forces HA and VA do not do any virtual work
| Sr. no. | Active force | Co-ordinate of the point of action along the force | Virtual displacement |
| 1. | W = 25N | 0.2sinθ | 𝛿yB=0.2cosθ 𝛿θ |
| 2. | 40 N | 0.3sinθ | 𝛿yC=0.3cosθ 𝛿θ |
| 3. | T | 0.4sinθ | 𝛿yD=0.4cos θ𝛿θ |
By using the principle of virtual work
, 𝛿U=0
-25 x 𝛿yB -40 x 𝛿yC +T x 𝛿yD=0
T x 𝛿yD = 25 x 𝛿yB + 40 x 𝛿yC
T x (0.4cos θ𝛿θ) = 25 x (0.2cosθ 𝛿θ) + 40 x (0.3cosθ 𝛿θ) Dividing by cosθ 𝛿θ and solving
T=42.5N
Tension in the cable=42.5N
APPEARS IN
संबंधित प्रश्न
Find the support reactions at A and B for the beam loaded as shown in the given figure.
State Lami’s theorem.
State the necessary condition for application of Lami’s theorem.
Three blocks A,B and C of masses 3 kg,2 kg and 7 kg respectively are connected as shown.Determine the acceleration of A,B and C.Also find the tension in the string.
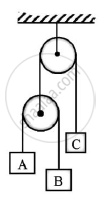
Given : mA=3kg
mB=2 kg
mC=7kg
To find: Acceleration of blocks A,B and C
Block A of weight 2000N is kept on the inclined plane at 35° .It is connected to weight B by an inextensible string passing over smooth pulley.
Determine the weight of pan B so that B just moves down.Assume μ=0.2.
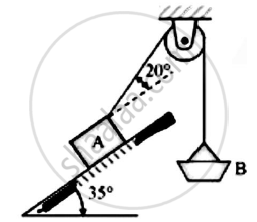
Given : Weight of block A=2000N
Angle of inclined plane = 35°
μ=0.2
To find : Weight of pan B
