Advertisements
Advertisements
प्रश्न
A uniform disc of radius R, is resting on a table on its rim.The coefficient of friction between disc and table is µ (Figure). Now the disc is pulled with a force F as shown in the figure. What is the maximum value of F for which the disc rolls without slipping?

उत्तर
Let the acceleration of the centre of mass of the disc be ‘a’, then
`Ma = F - f` ......(1)
The angular acceleration of the disc is `α = a/R`. (if there is no sliding).
Then `(1/2 MR^2)α = Rf` ......(2)
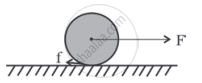
⇒ `Ma = 2f`
Thus, `f = F/3`. Since there is no sliding,
⇒ `f ≤ µmg`
⇒ `F ≤ 3µMg`.
APPEARS IN
संबंधित प्रश्न
Read each statement below carefully, and state, with reasons, if it is true or false;
For perfect rolling motion, work done against friction is zero.
Read each statement below carefully, and state, with reasons, if it is true or false;
A wheel moving down a perfectly frictionless inclined plane will undergo slipping (not rolling) motion
If a rigid body of radius ‘R’ starts from rest and rolls down an inclined plane of inclination
‘θ’ then linear acceleration of body rolling down the plane is _______.
Two uniform solid spheres having unequal masses and unequal radii are released from rest from the same height on a rough incline. If the spheres roll without slipping, ___________ .
Discuss the interlink between translational, rotational and total kinetic energies of a rigid object rolls without slipping.
Answer in Brief:
A rigid object is rolling down an inclined plane derive the expression for the acceleration along the track and the speed after falling through a certain vertical distance.
An object is rolling without slipping on a horizontal surface and its rotational kinetic energy is two-thirds of translational kinetic energy. The body is ______.
The kinetic energy and angular momentum of a body rotating with constant angular velocity are E and L. What does `(2E)/L` represent?
When a sphere rolls without slipping, the ratio of its kinetic energy of translation to its total kinetic energy is ______.
A disc of mass 4 kg rolls on a horizontal surface. If its linear speed is 3 m/ s, what is its total kinetic energy?
