Question

Akhila went to a fair in her village. She wanted to enjoy rides in the Giant Wheel and play Hoopla (a game in which you throw a rig on the items kept in the stall, and if the ring covers any object completely you get it.) The number of times she played Hoopla is half the number of rides she had on the Giant Wheel. Each ride costs Rs 3, and a game of Hoopla costs Rs 4. If she spent Rs 20 in the fair, represent this situation algebraically and graphically.

Answer 1

The pair of equations formed is:

`y - 1/x `

i.e x - 2y = 0   ....(1)

3x + 4y = 20 ....(2)

Let us represent these equations graphically. For this, we need at least two solutions for each equation. We give these solutions in Table

x	0	2
`y - x/2`	0	1

 

x	0	2	4
y = `(20-3x)/4`	5	0	2

Recall from Class IX that there are infinitely many solutions of each linear equation. So each of you chooses any two values, which may not be the ones we have chosen. Can you guess why we have chosen xO in the first equation and in the second equation? When one of the variables is zero, the equation reduces to a linear equation is one variable, which can be solved easily. For instance, putting x O in Equation (2), we get 4y = 20 i.e. y = 5. Similarly, putting yO in Equation (2), we get 3x = 20 i.e  `x = 20/3`, But as  `20/3`is not an integer. it will not be easy to plot exactly on the graph paper. So, we choose y = 2 which gives x = 4, an integral value.

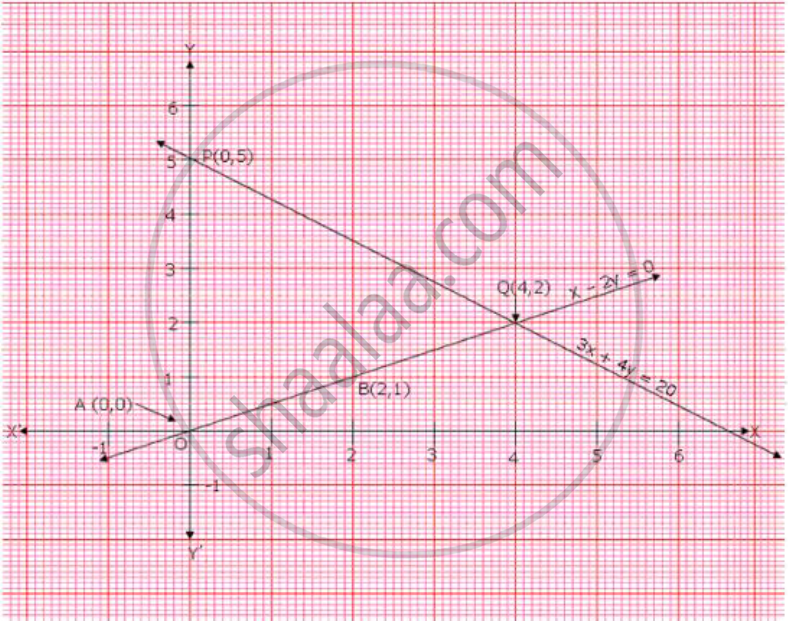
Plot the points A(O, O), B(2,1) and P(O,5), Q(4,2) corresponding to the draw the lines AB and PQ, representing the equations x - 2y = 0 and 3x +  4y = 20, as shown in figure

In fig., observe that the two lines representing the two equations are intersecting at the point (4,2),