Question

An electrician has to repair an electric fault on a pole of height 4 m. He needs to reach a point 1.3 m below the top of the pole to undertake the repair work. What should be the length of the ladder that he should use which when inclined at an angle of 60° to the horizontal would enable him to reach the required position?

विकल्प

• `(9 sqrt3)/5`

• `(9 xx 5)/sqrt3`

• `9/sqrt3`

• `sqrt3/5`

Answer 1

`(9 sqrt3)/5`

Explanation:

Let AC be the electric pole of height 4 m. Let B be a point 1.3 m below the top A of the pole AC.

Then, BC = AC – AB = (4 – 1.3) m = 2.7 m

Let BD be the ladder inclined at an angle of 60° to the horizontal.

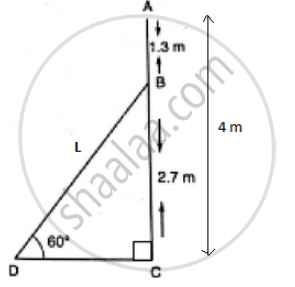
In ΔBCD, we have

`"sin"  60^circ = 2.7/"L"`

`=> sqrt3/2 = 2.7/"L"     [because "BC" = 2.7  "m"]`

`sqrt3/2 = 2.7/"L"`

`"L" = 2/sqrt3 ((27))/10`

`= 1.8 sqrt3  "m"`

`"or"  9/5 sqrt3  "m"`

`=> "BD" = (2 xx 2.7)/sqrt3  "m"`

`= 5.4 /sqrt3  "m"`

`= (5.4 xx sqrt3)/3  "m"`

`=> "BD" = (1.8) sqrt3  "m" = 9/5 sqrt 3  "m"`