Question

An inclined plane is bent in such a way that the vertical cross-section is given by Y = `x^2/4` where y is in vertical and x in horizontal direction. If the upper surface of this curved plane is rough with coefficient of friction µ = 0.5, the maximum height in cm at which a stationary block will not slip downward is ______ cm.

विकल्प

• 22

• 23

• 25

• 26

Answer 1

An inclined plane is bent in such a way that the vertical cross-section is given by Y = `x^2/4` where y is in vertical and x in horizontal direction. If the upper surface of this curved plane is rough with coefficient of friction µ = 0.5, the maximum height in cm at which a stationary block will not slip downward is 25 cm.

Explanation:

Given, equation of vertical cross-section

Y= `x^2/4`

Coefficient of friction (µ) = 0.5

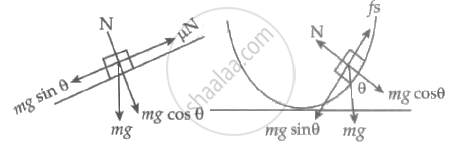
Now, applying the force balance equation,

mg sinθ = µ N = f_s 

⇒ mg sinθ = µ mg cosθ

⇒ tanθ = µ            ... (i)

Now, y = `x^2/4`

 tanθ = `"dy"/("d"x)`

= `(2x)/4`

= `x/2`

From equation (i)

⇒ tanθ = µ `x/2`

⇒ x = 2µ = 2 × 0.5

⇒ x = 1

Hence, y = `x^2/4`

y = `1/4`

y = 0.25 cm

or Y = 25 cm.