Advertisements
Advertisements
प्रश्न
Factorise the following using suitable identity
a2 + 6ab + 9b2 – c2
उत्तर
a2 + 6ab + 9b2 – c2 = a2 + 2 × a × 3b + 32b2 – c2
= a2 + (2 × a × 3b) + (3b)2 – c2
This expression is of the form of identity
[a2 + 2ab + b2] – c2 = (a + b)2 – c2
a2 + (2 × a × 3b) + (3b)2 – c2 = (a + 3b)2 – c2
Again this R.H.S is of the form of identity
a2 – b2 = (a + b)(a – b)
(a + 3b)2 – c2 = [(a + 3b) + c][(a + 3b) – c]
a2 + 6ab + 9b2 – c2 = (a + 3b + c)(a + 3b – c)
APPEARS IN
संबंधित प्रश्न
Use a suitable identity to get the following products.
(x + 3) (x + 3)
Use a suitable identity to get the following products.
(7a − 9b) (7a − 9b)
Use the formula to find the value.
54 × 46
Expand: (10 + y)2
(x2 + y2)(y2 + x2) = (x2 + y2)2
Factorise the following, using the identity a2 + 2ab + b2 = (a + b)2.
`x^2/4 + 2x + 4`
The area of a square is 9x2 + 24xy + 16y2. Find the side of the square.
If p + q = 12 and pq = 22, then find p2 + q2.
Verify the following:
(7p – 13q)2 + 364pq = (7p + 13q)2
Take suitable number of cards given in the adjoining diagram [G(x × x) representing x2, R(x × 1) representing x and Y(1 × 1) representing 1] to factorise the following expressions, by arranging the cards in the form of rectangles:
- 2x2 + 6x + 4
- x2 + 4x + 4.
Factorise 2x2 + 6x + 4 by using the figure.
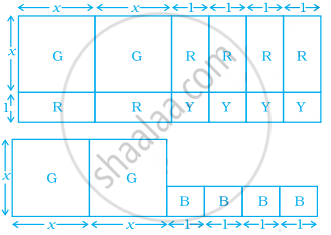
Calculate the area of figure.
