Advertisements
Advertisements
प्रश्न
For the following inequation, represent the solution on a number line :
3(2x -1) ≥ 2(2x + 3), x ∈ Z
उत्तर
3(2x -1) ≥ 2(2x + 3), x ∈ Z
⇒ 6x - 3 ≥ 4x + 6, x ∈ Z
⇒ 6x - 4x ≥ 6 + 3
⇒ 2x ≥ 9
⇒ x ≥
⇒ x ≥
∴ x = {5, 6, 7, ....}
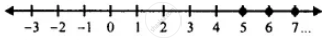
APPEARS IN
संबंधित प्रश्न
Solve and graph the solution set on a number line: 5x + 4 > 8x – 11 ; x ∈ Z
Solve and graph the solution set on a number line:
For the following inequation, represent the solution on a number line :
2(4 - 3x) ≤ 4(x - 5), x ∈ W
For the following inequation, represent the solution on a number line :
4(3x + 1) > 2(4x - 1), x is a negative integer
Fill in the blanks, using the following number line:
2 is _______ than – 4 implies 2 is to the ________ of – 4.
Fill in the blanks, using the following number line:

-3 is _________ than 2 and 3 is _______ than – 2.
Fill in the blanks, using the following number line:

5 is _______ than 2 and -5 is ______ than – 2.
Fill in the blanks, using the following number line:

8 is _______ than -5 and -8 is ______than -5.
In the following case, arrange the given integers in ascending order using a number line.
– 8, 0, – 5, 5, 4, – 1
For the statement, given below, state whether it is true or false:
Every negative integar is smaller than 0.
