Advertisements
Advertisements
प्रश्न
If a perpendicular is drawn from the vertex containing the right angle of a right triangle to the hypotenuse then prove that the triangle on each side of the perpendicular are similar to each other and to the original triangle. Also, prove that the square of the perpendicular is equal to the product of the lengths of the two parts of the hypotenuse
उत्तर
Given: A right triangle ABC right angled at B, BD ⊥ AC.
To Prove:
(i) ∆ADB ~ ∆BDC (ii) ∆ADB ~ ∆ABC
(iii) ∆BDC ~ ∆ABC (iv) BD2 = AD × DC
(v) AB2 = AD × AC (vi) BC2 = CD × AC
Proof:
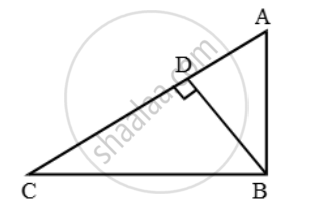
(i) We have,
∠ABD + ∠DBC = 90º
Also, ∠C + ∠DBC + ∠BDC = 180º
⇒ ∠C + ∠DBC + 90º = 180º
⇒ ∠C + ∠DBC = 90º
But, ∠ABD + ∠DBC = 90º
∴ ∠ABD + ∠DBC = ∠C + ∠DBC
⇒ ∠ABD = ∠C ….(i)
Thus, in ∆ADB and ∆BDC, we have ∠ABD = ∠C [From (i)] and, ∠ADB = ∠BDC [Each equal to 90º]
So, by AA-similarity criterion, we have
∆ADB ~ ∆BDC
(ii) In ∆ADB and ∆ABC, we have
∠ADB = ∠ABC [Each equal to 90º] and, ∠A = ∠A [Common]
So, by AA-similarity criterion, we have
∆ADB ~ ∆ABC
(iii)In ∆BDC and ∆ABC, we have
∠BDC = ∠ABC [Each equal to 90º]
∠C = ∠C [Common]
So, by AA-similarity criterion, we have
∆BDC ~ ∆ABC
(iv) From (i), we have
∆ADB ~ ∆BDC
`\Rightarrow \frac{AD}{BD}=\frac{BD}{DC}`
`⇒ BD^2 = AD × DC`
(v) From (ii), we have
`\Rightarrow \frac{AD}{AB}=\frac{AB}{AC}`
∆ADB ~ ∆ABC
⇒ AB2 = AD × AC
(vi) From (iii), we have
`\Rightarrow \frac{BC}{AC}=\frac{DC}{BC}`
∆BDC ~ ∆ABC
⇒ BC2 = CD × AC
