Advertisements
Advertisements
प्रश्न
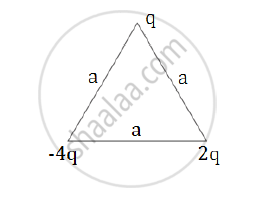
Obtain an expression for the work done to dissociate the system of three charges placed at the vertices of an equilateral triangle of side ‘a’ as shown below.
उत्तर
Work done to keep the system bound is
`W=(Kq_1q_2)/a+(Kq_2q_3)/a+(Kq_1q_3)/a`
`W=(Kq(-4q))/a+(K(-4q)2q)/a+(Kq(2q))/a`
`W=(-K4q^2)/a-(K8q^2)/a+(K2q^2)/a`
`W=(Kq^2)/a(-4-8+2)=(-10Kq^2)/a`
Therefore, the work done to dissociate the system is
`W_d=-W=(10Kq^2)/a`
APPEARS IN
संबंधित प्रश्न
What is the force between two small charged spheres having charges of 2 × 10−7 C and 3 × 10−7 C placed 30 cm apart in air?
The force between two small charged spheres having charges of 1 × 10−7 C and 2 × 10−7 C placed 20 cm apart in the air is:
Three charges each equal to 1 µC are placed at the comers of an equilateral triangle. If the force between any two charges is F, then the net force on either will be ______
