Question

Find the smallest number by which 1152 must be divided so that it becomes a perfect square. Also, find the number whose square is the resulting number.

Answer 1

Prime factorisation of 1152:
1152 = 2 x 2 x 2 x 2 x 2 x 2 x 2 x 3 x 3

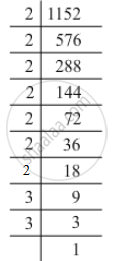
Grouping them into pairs of equal factors:
1152 = (2 x 2) x (2 x 2) x (2 x 2) x (3 x 3) x 2
The factor, 2 at the end is not paired. For a number to be a perfect square, each prime factor has to be paired. Hence, 1152 must be divided by 2 for it to be a perfect square.
The resulting number would be (2 x 2) x (2 x 2) x (2 x 2) x (3 x 3).
Furthermore, we have:
(2 x 2) x (2 x 2) x (2 x 2) x (2 x 2) x (3 x 3) = (2 x 2 x 2 x 3) x (2 x 2 x 2 x 3)
Hence, the number whose square is the resulting number is:
2 x 2 x 2 x 3 = 24