Question

Places A and B are 180 km apart on a highway. One car starts from A and another from B at the same time. If the car travels in the same direction at different speeds, they meet in 9 hours. If they travel towards each other with the same speeds as before, they meet in an hour. What are the speeds of the two cars?

Answer 1

Let the speed of the first car be x km/hr and the speed of the second car be y km/hr.

If travelling in the same direction

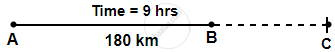
Distance travelled by 1^st car = AC = AB + BC

Distance travelled by 2^nd car = BC

Difference of distance travelled = (AB + BC) − BC = AB = 180 km

Distance travelled by 1^st car − Distance travelled by 2^nd car = 180 km

(Speed of 1^st car × 9 hours) − (Speed of 2^nd car × 9 hours) = 180 km

9x − 9y = 180

9(x − y) = 180

(x − y) = `180/9`

x − y = 20  ...(1)

If travelling in the opposite direction

Distance travelled by 1^st car = AD

Distance travelled by 2^nd car = BD

The sum of distance travelled = AD + BD = AB = 180 km

Distance travelled by 1^st car + Distance travelled by 2^nd car = 180 km

(Speed of 1^st car × 1 hour) + (Speed of 2^nd car × 1 hour) = 180 km

x + y = 180 ...(2)

So, our two equations are

x − y = 20  ...(1)

x + y = 180 ...(2)

From (1)

x − y = 20

x = 20 + y

Putting the value of x in equation (2)

x + y = 180

20 + y + y = 180

20 + 2y = 180

2y = 180 − 20

2y = 160

y = `160/2`

∴ y = 80

Putting y = 80 in equation (1)

x − y = 20

X − 80 = 20

x = 20 + 80

X = 100

Therefore, x = 100, y = 80 is the solution.

Thus,

Speed of first car = x km/h = 100 km/hr.

Speed of second car = y km/h = 80 km/hr.