Advertisements
Advertisements
प्रश्न
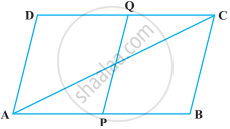
Points P and Q have been taken on opposite sides AB and CD, respectively of a parallelogram ABCD such that AP = CQ (Figure). Show that AC and PQ bisect each other.
उत्तर
Given: ABCD is a parallelogram and AP = CQ
To show: AC and PQ bisect each other.
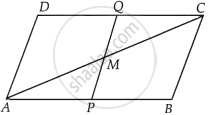
Proof: In ΔAMP and ΔCMQ,
∠MAP = ∠MCQ ...[Alternate interior angles]
AP = CQ ...[Given]
And ∠APM = ∠CQM ...[Alternate interior angles]
∴ ΔAMP ≅ ΔCMQ ...[By ASA congruence rule]
⇒ AM = CM ...[By CPCT rule]
And PM = MQ ...[By CPCT rule]
Hence, AC and PQ bisect each other.
Hence proved.
APPEARS IN
संबंधित प्रश्न
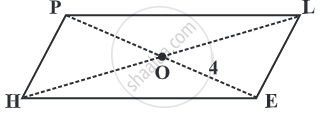
Diagonals AC and BD of a parallelogram ABCD intersect each other at O. If OA = 3 cm and OD = 2 cm, determine the lengths of AC and BD.
In a parallelogram ABCD, AB = 10 cm and AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.
A diagonal of a parallelogram bisects one of its angles. Show that it is a rhombus.
In the following figure, AB || DE, AB = DE, AC || DF and AC = DF. Prove that BC || EF and BC = EF.

P and Q are points on opposite sides AD and BC of a parallelogram ABCD such that PQ passes through the point of intersection O of its diagonals AC and BD. Show that PQ is bisected at O.
ABCD is a rectangle in which diagonal BD bisects ∠B. Show that ABCD is a square.
P is the mid-point of the side CD of a parallelogram ABCD. A line through C parallel to PA intersects AB at Q and DA produced at R. Prove that DA = AR and CQ = QR.
The point of intersection of diagonals of a quadrilateral divides one diagonal in the ratio 1:2. Can it be a parallelogram? Why or why not?
Two sticks each of length 5 cm are crossing each other such that they bisect each other. What shape is formed by joining their endpoints? Give reason.
