Question

Prove geometrically that when plane mirror turns through a certain angle, the reflected ray turns through twice the angle.

Answer 1

Consider a ray of light AB, incident on a plane mirror in position MM’, such that BC is the reflected ray and BN is normal.

∠ABM = ∠CBN = ∠i
∠ABC = 2 ∠i …(i)

Let the mirror be rotated through an angle ‘0’ about point B, such that M₁M₁ is the new position of the mirror and BN₁ is the new position of normal. As the position of the incident ray remains same, therefore new angle of the incidence is ∠ABN₁ whose magnitude is (i + θ). Let BD be the reflected ray, such that ∠DNB₁ is the new angle of reflection.

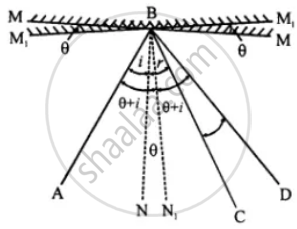
∠ABD = ∠ABN₁ + ∠DBN₁

= ∠(i + θ) + ∠(i + θ)

= 2 ∠i + 2∠θ

Subtracting (i) from (ii)

∠ABD - ∠ABC = 2∠i + 2∠θ - 2∠i

∴ ∠CBD = 2∠θ

Thus, for a given incident ray, if the plane mirror is rotated through a certain angle, then the reflected ray rotates through twice the angle.