Advertisements
Advertisements
प्रश्न
The area of a rectangular field is 1600 sq m. If the length of the field is 80 m, find the perimeter of the field.
उत्तर
Given, area of the rectangular field = 1600 sq m
And length of the rectangular field = 80 m
We know that, area of rectangle = Length × Breadth
Let breadth of the rectangular field = b
Then, Area = 80 × b
⇒ 1600 = 80 × b
⇒ `1600/80 = (80 xx b)/80` .....[Dividing both sides by 80]
⇒ b = 20 m
Now, perimeter of the rectangular field = 2 × (Length + Breadth)
= 2 × (80 + 20)
= 2 × 100
= 200 m
APPEARS IN
संबंधित प्रश्न
A rectangular field has length = 160m and breadth = 120 m. Find:
(i) the perimeter of the field.
(ii) the length of fence required to enclose the field.
(iii) the cost of fencing the field at the rate of? 80 per meter.
If A denotes area of a rectangle, l represents its length and b represents its breadth, find:
l, if A = 48 cm² and b = 6 cm
If A denotes area of a rectangle, l represents its length and b represents its breadth, find:
b, if A = 88 m² and l = 8m
The table given below contains some measures of the rectangle. Find the unknown values.
| Length | Breadth | Perimeter | Area |
| 5 cm | 8 cm | ? | ? |
If every side of a rectangle is doubled, then its area becomes _______ times
How many different rectangles can be made with a 48 cm long string? Find the possible pairs of length and breadth of the rectangles.
Find the perimeter of the following figure
Length of a rectangle is three times its breadth. Perimeter of the rectangle is 40 cm. Find its length and width.
There is a rectangular lawn 10m long and 4m wide in front of Meena’s house (see the figure). It is fenced along the two smaller sides and one longer side leaving a gap of 1m for the entrance. Find the length of fencing.
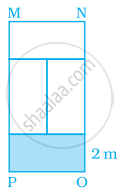
Rectangle MNOP is made up of four congruent rectangles (Figure). If the area of one of the rectangles is 8 m2 and breadth is 2 m, then find the perimeter of MNOP.