Advertisements
Advertisements
प्रश्न
The external dimensions of a closed wooden box are 48 cm, 36 cm, 30 cm. The box is made of 1.5 cm thick wood. How many bricks of size 6 cm x 3 cm x 0.75 cm can be put in this box?
उत्तर
Given internal dimensions are
`l=48-2xx"thickness"=48-3=45cm`
`b=36-3=33cm`
`h=30-3=27cm`
∴ Internal volume =`45xx33xx27cm^3`
Volume of brick = `5xx3xx0.75cm^3`
Hence, number of bricks= `"Internal volume"/"volume of 1 brick"`
`=(45xx33xx27)/(6xx3xx0.37)`
`=(38880)/13.5`
`=2970`
`∴2970 "bricks can be kept inside the box"`
APPEARS IN
संबंधित प्रश्न
A cuboidal vessel is 10 m long and 8 m wide. How high must it be made to hold 380 cubic metres of a liquid?
If V is the volume of a cuboid of dimensions a, b, c and S is its surface area, then prove that
`1/V=2/S(1/a+1/b+1)`
A wall of length 10 m was to be built across an open ground. The height of the wall is 4 m and thickness of the wall is 24 cm. If this wall is to be built up with bricks whose dimensions are 24 cm × 12 cm × 8 cm, How many bricks would be required?
The number of bricks each measuring 50 cm × 30 cm × 20 cm that will be required to build a wall whose dimensions are 5 m × 3 m × 2 m is
Volume of regular solids such as cube and cuboid can be found by multiplying the dimensions. Complete the given table by finding the volume of the given objects
| S.No. | Objects | l | b | h | Volume (cubic cm) |
| 1. | Note books | 6 cm | 15 cm | 1 cm | |
| 2. | Name board | 20 cm | 90 cm | 2 cm | |
| 3. | Show case cub board | 70 cm | 250 cm | 70 cm | |
| 4. | Gift box | 10 cm | 10 cm | 10 cm | |
| 5. | Dice | 1 cm | 1 cm | 1 cm |
The surface area of the three coterminus faces of a cuboid are 6, 15 and 10 cm2 respectively. The volume of the cuboid is ______.
A truck carrying 7.8 m3 concrete arrives at a job site. A platform of width 5 m and height 2 m is being contructed at the site. Find the length of the platform, constructed from the amount of concrete on the truck?
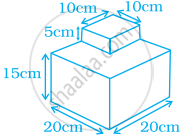
A birthday cake has two tiers as shown in the figure below. Find the volume of the cake.