Question

The normal to the ellipse `x^2/a^2 + y^2/b^2` = 1 at a point P(x₁, y₁) on it, meets the x-axis in G. PN is perpendicular to OX, where O is origin. Then value of ℓ(OG)/ℓ(ON) is ______.

विकल्प

• e

• e²

• e³

• 1 – e²

Answer 1

The normal to the ellipse `x^2/a^2 + y^2/b^2` = 1 at a point P(x₁, y₁) on it, meets the x-axis in G. PN is perpendicular to OX, where O is origin. Then value of ℓ(OG)/ℓ(ON) is `underlinebb(e^2)`.

Explanation:

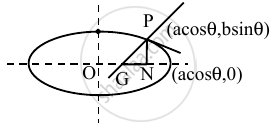
Normal to the ellipse: `(ax)/cosθ - (by)/sinθ` = a² – b²

`(ax)/cosθ - (by)/sinθ` = a²e²

`G((cosθ(a^2.e^2))/a, 0)`

N(a cosθ, 0)

`(ℓ(OG))/(ℓ(ON)) = (ae^2cosθ)/(acosθ)` = e²