Advertisements
Advertisements
प्रश्न
The perimeter of a square plot = 360 m. Find:
- its area.
- cost of fencing its boundary at the rate of ₹ 40 per meter.
- cost of levelling the plot at ₹60 per square meter.
उत्तर
Given the perimeter of the square plot = 360 m
Side Length of the Square: `"Side Length" = "Perimeter"/4 = 360/4 = 90 m`
Area of the Square: Area = (Side Length)2 = 902 = 8100 m2
Cost of Fencing: The cost of fencing is calculated as:
Cost of Fencing = Perimeter × Rate per meter.
Cost of Fencing = 360 × 40 = ₹ 14,400.
Cost of Levelling = Area × Rate per square meter.
Substituting the values: Cost of Levelling = 8100 × 60 = ₹ 4,86,000.
- Area of the plot = 8100 m2
- Cost of fencing = ₹ 14,400
- Cost of levelling = ₹ 4,86,000
APPEARS IN
संबंधित प्रश्न
The length of a rectangle is 16 cm and its perimeter is equal to the perimeter of a square with side 12.5 cm. Find the area of the rectangle.
A path of uniform width, 2.5 m, runs around the inside of a rectangular field 30 m by 27 m. Find the area of the path.
The side of a square is 3.6 cm; find its perimeter.
How many different rectangles can be made with a 48 cm long string? Find the possible pairs of length and breadth of the rectangles.

Each rectangle is made out of 12 equal squares, so all have the same area, but the length of the boundary will be different.
Which of these rectangles has the longest perimeter?
Length of a rectangle is three times its breadth. Perimeter of the rectangle is 40 cm. Find its length and width.
There is a rectangular lawn 10m long and 4m wide in front of Meena’s house (see the figure). It is fenced along the two smaller sides and one longer side leaving a gap of 1m for the entrance. Find the length of fencing.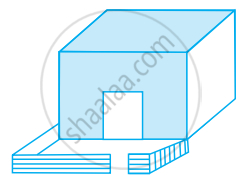
36 unit squares are joined to form a rectangle with the least perimeter. Perimeter of the rectangle is ______.
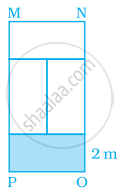
Rectangle MNOP is made up of four congruent rectangles (Figure). If the area of one of the rectangles is 8 m2 and breadth is 2 m, then find the perimeter of MNOP.
A large square is made by arranging a small square surrounded by four congruent rectangles as shown in the given figure. If the perimeter of each of the rectangle is 16 cm, find the area of the large square.