Advertisements
Advertisements
प्रश्न
The reciprocal of `(2/5)^-1` is ______.
विकल्प
`2/5`
`5/2`
`- 5/2`
` - 2/5`
उत्तर
The reciprocal of `(2/5)^-1` is `underlinebb(5/2)`.
Explanation:
Using law of exponents, `a^-m = 1/a^m` ...[∵ A is non-zero integer]
∴ `(2/5)^-1 = 1/(2/5)^1 = 5/2`
APPEARS IN
संबंधित प्रश्न
If y be any non-zero integer, then y0 is equal to ______.
`((-7)/5)^-1` is equal to ______.
`10^-2 = 1/100`
`(2/3)^-2 xx (2/3)^-5 = (2/3)^10`
`(-8/2)^0 = 0`
`a^m = 1/a^-m`
Find a single repeater machine that will do the same work as hook-up.
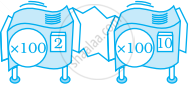
If possible, find a hook-up of prime base number machine that will do the same work as the given stretching machine. Do not use (× 1) machines.

The left column of the chart lists the lengths of input chains of gold. Repeater machines are listed across the top. The other entries are the outputs you get when you send the input chain from that row through the repeater machine from that column. Copy and complete the chart.
| Input Length | Repeater Machine | ||
| × 23 | |||
| 40 | 125 | ||
| 2 | |||
| 162 | |||
Simplify:
`(4/13)^4 xx (13/7)^2 xx (7/4)^3`
