Advertisements
Advertisements
प्रश्न
The sum of a two-digit number and the number obtained by reversing the digits is always divisible by ______.
उत्तर
The sum of a two-digit number and the number obtained by reversing the digits is always divisible by 11.
Explanation:
Let ab be any two-digit number, then the number obtained by reversing its digits is ba.
Now, ab + ba = (10a + b) + (10b + a)
= 11a + 11b
= 11(a + b)
Hence, ab + ba is always divisible by 11 and (a + b).
APPEARS IN
संबंधित प्रश्न
Find the quotient when the difference of 985 and 958 is divided by 9.
Which of the following statement is true?
If a number is divisible by 8, it must be divisible by 4.
Which of the following statement is true?
If a number is divisible by both 9 and 10, it must be divisible by 90.
Do you remember magic triangles? Come now, let s make some magic squares.
- Fill this square using all the numbers from 46 to 54.
Rule: The total of each line is 150.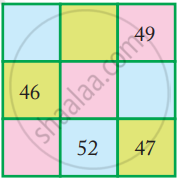
The difference of a two-digit number and the number obtained by reversing its digits is always divisible by ______.
A three-digit number abc is divisible by 6 if c is an even number and a + b + c is a multiple of 3.
Number 7N + 1 will leave remainder 1 when divided by 7.
If AB + 7C = 102, where B ≠ 0, C ≠ 0, then A + B + C = 14.
If N ÷ 5 leaves remainder 3 and N ÷ 2 leaves remainder 0, then N ÷ 10 leaves remainder 4.
Fill in the blank space in the same way.

