Advertisements
Advertisements
प्रश्न
The taxi charges in a city comprised of a fixed charge of ₹ 100 for 5 kms and ₹ 16 per km for every additional km. If the amount paid at the end of the trip was ₹ 740 find the distance travelled.
उत्तर
Let the distance travelled by taxi be ‘x’ km
For the first 5 km the charge = ₹ 100
For additional kms the charge = ₹ 16(x – 5)
∴ For x kms the charge = 100 + 16(x – 5)
Amount paid = ₹ 740
∴ 100 + 16(x – 5) = 740
100 + 16(x – 5) – 100 = 740 – 100
16(x – 5) = 640
`(16(x - 5))/16 = 640/16`
x – 5 = 40
x – 5 + 5 = 45 + 5
x = 45
x = 45 km
∴ Total distance travelled = 45 km
APPEARS IN
संबंधित प्रश्न
Find x: −3(4x + 9) = 21
x = 25 is the solution of one third of a number is less than 10 the original number.
How much smaller is 2ab + 4b – c than 5ab – 3b + 2c
A piece of wire is ‘12s’ cm long. What will be the length of the side, if it is formed as an equilateral triangle
Gopal is 8 years younger to Karnan. If the sum of their ages is 30, how old is Karnan?
The rectangles made of identical square blocks with varying lengths but having only two square blocks as width are given below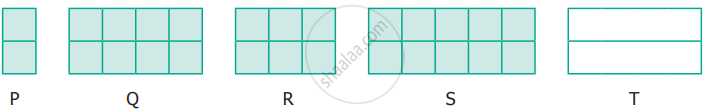
(i) How many small size squares are there in each of the rectangles P, Q, R and S?
(ii) Fill in the boxes
| Rectangle | P | Q | R | S | T |
| Number of small size squares along the breadth | 2 | 2 | ? | 2 | 2 |
| Number of squares along the length | 1 | 4 | 3 | ? | x |
| Total number of squares in rectangle | ? | 8 | ? | 10 | ? |
If the perimeter of a regular hexagon is x metres, then the length of each of its sides is ______.
`q/2` = 3 has a solution ______.
a = 3 is a solution of the equation 2a – 1 = 5.
| Column I | Column II |
| (i) The number of corners of a quadrilateral | (A) = |
| (ii) The variable in the equation 2p + 3 = 5 | (B) constant |
| (iii) The solution of the equation x + 2 = 3 | (C) +1 |
| (iv) solution of the equation 2p + 3 = 5 | (D) –1 |
| (v) A sign used in an equation | (E) p |
| (F) x |
