Advertisements
Advertisements
प्रश्न
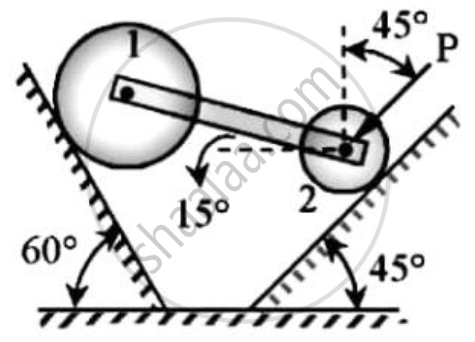
Two cylinders 1 and 2 are connected by a rigid bar of negligible weight hinged to each cylinder and left to rest in equilibrium in the position shown under the application of force P applied at the center of cylinder 2.
Determine the magnitude of force P.If the weights of the cylinders 1 and 2 are 100N and 50 N respectively.
उत्तर
Given : W1 = 100 N
W2 = 50 N
Cylinders are connected by a rigid bar
To find : Magnitude of force P
Solution :
Consider cylinder I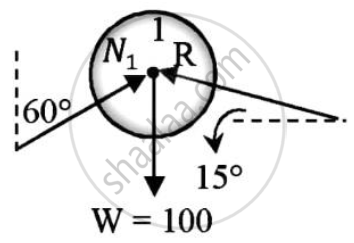
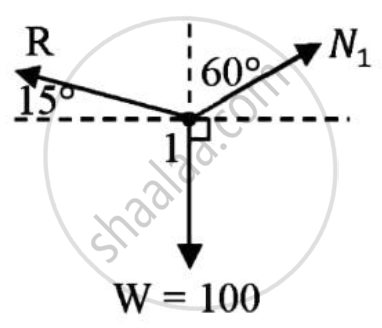
Applying Lami’s theorem :
`R/(sin(90+30))=W/sin(60+75)=N_1/sin(90+15)`
R=`(100)/(sin135)Xsin120`
R = 122.4745 N
Cylinder 2 is under equilibrium
Applying conditions of equilibrium
ΣFy = 0
N2sin45 - Rsin15 - Psin45 – W = 0
N2sin45 - Psin45 = 122.4745 x 0.2588 + 50
N2sin45 - Psin45 = 81.6987 ………..(1)
Applying conditions of equilibrium
ΣFx = 0
-N2cos45+Rcos15-Pcos45=0
N2cos45+Pcos 45=118.3013 ……(2)
Solving (1) and (2)
P=25.8819 N
Magnitude of force P required = 25.8819 N
APPEARS IN
संबंधित प्रश्न
Determine the tension in a cable BC shown in fig by virtual work method.

Given: F=3500 N
ϴ = 50o
Length of rod = 3.75 mm + 1.5 mm = 5.25 mm
To find : Tension in cable BC
Determine the required stiffness k so that the uniform 7 kg bar AC is in equilibrium when θ=30o.
Due to the collar guide at B the spring remains vertical and is unstretched when θ = 0o.Use principle of virtual work.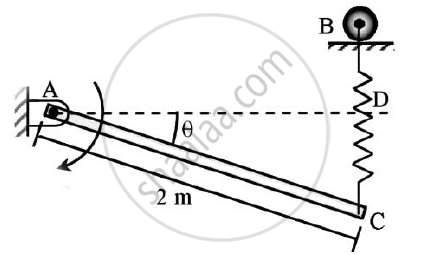
Using Principle of Virtual Work, determine the force P which will keep the weightless bar AB in equilibrium. Take length AB as 2m and length AC as 8m. The bar makes an angle of 30° with horizontal. All the surfaces in contact are smooth. Refer Figure 9.