Advertisements
Advertisements
प्रश्न
Use an expansion formula to find the value.
(997)2
उत्तर
It is known that, (a + b)2 = a2 + 2ab + b2 and (a − b)2 = a2 − 2ab + b2
(997)2
= (1000 − 3)2
= (1000)2 − 2 × 1000 × 3 + (3)2
= 1000000 − 6000 + 9
= 994009
संबंधित प्रश्न
Using identities, evaluate 297 × 303
Expand: (10 + y)2
Expand: `("y" - 3/"y")^2`
Expand: (51)2
Expand (3m + 5)2
If a + b = 10 and ab = 18, find the value of a2 + b2
Show that (x + 2y)2 – (x – 2y)2 = 8xy
Factorise the following, using the identity a2 + 2ab + b2 = (a + b)2.
4x2 + 12x + 9
Factorise the following, using the identity a2 + 2ab + b2 = (a + b)2.
9x2 + 30x + 25
Take suitable number of cards given in the adjoining diagram [G(x × x) representing x2, R(x × 1) representing x and Y(1 × 1) representing 1] to factorise the following expressions, by arranging the cards in the form of rectangles: x2 + 4x + 4. Factorise 2x2 + 6x + 4 by using the figure.
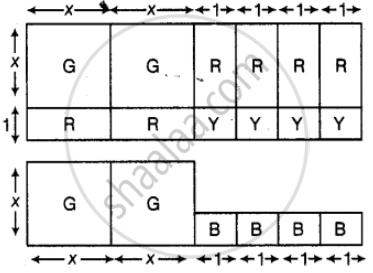
Calculate the area of figure.
