Advertisements
Advertisements
प्रश्न
Use rule and compass only for answering this question.
Draw a circle of radius 4 cm. Mark the centre as O. Mark a point P outside the circle at a distance of 7 cm from the centre. Construct two tangents to the circle from the external point P.
Measure and write down the length of any one tangent.
उत्तर
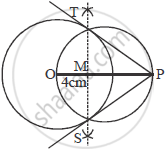
i. Draw a line segment OP = 7 cm
ii. With centre O and radius 4 cm, draw a circle.
iii. Draw the mid point of OP.
iv. With centre M and diameter OP, draw a circle which intersect the circle at T and S.
v. Joint PT and PS
PT and PS are the required tangent on measuring the length of PT = PS = 5.74 cm
APPEARS IN
संबंधित प्रश्न
Draw two concentric circles of radii 3 cm and 5 cm. Construct a tangent to smaller circle from a point on the larger circle. Also measure its length.
Draw a line AB = 5 cm. Mark a point C on AB such that AC = 3 cm. Using a ruler and a compass only, construct :
- A circle of radius 2.5 cm, passing through A and C.
- Construct two tangents to the circle from the external point B. Measure and record the length of the tangents.
In the figure given below, O is the centre of the circle and SP is a tangent. If ∠SRT = 65°,
find the value of x, y and z.

In the figure given below, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find:
1) AB.
2) the length of tangent PT.

Construct a circle, inscribing an equilateral triangle with side 5.6 cm.
Draw two tangents to a circle of radius 3.5 cm form a point P at a distance of 6.2 cm form its centre.
Draw a line segment AB of length 8 cm. Taking A as centre , draw a circle of radius 4 cm and taking B as centre , draw another circle of radius 3 cm. Construct tangents to each circle form the centre of the other circle.
Draw a circle of radius 4.2. Draw a pair of tangents to this circle inclined to each other at an angle of 45°
Which of the following is not true for a point P on the circle?
Using ruler and compass construct a triangle ABC in which AB = 6 cm, ∠BAC = 120° and AC = 5 cm. Construct a circle passing through A, B and C. Measure and write down the radius of the circle.
