Advertisements
Advertisements
प्रश्न
Use the mirror equation to show that an object placed between f and 2f of a concave mirror produces a real image beyond 2f.
उत्तर
We know for a concave mirror, f < 0 [negative] and u < 0 [negative]
2f < u < f
∴ `1/(2"f") > 1/"u" > 1/"f"`
or `(-1)/(2"f") < (-1)/"u" < (-1)/"f"`
or `1/"f" - 1/(2"f") < 1/"f" - 1/"u" < 1/"f" - 1/"f"`
or `1/(2"f") < 1/"v" < 0 ...[∵ 1/"f" - 1/"u" = 1/"v"]`
This implies that v < 0 forms an image on the left.
Also, 2f > v .....[∵ 2f and v are negative]
|2f| < |v|
So, the real image is formed beyond 2f.
APPEARS IN
संबंधित प्रश्न
Use the mirror equation to show that a convex mirror always produces a virtual image independent of the location of the object.
Use the mirror equation to deduce that the virtual image produced by a convex mirror is always diminished in size and is located between the focus and the pole.
Use the mirror equation to deduce that an object placed between the pole and focus of a concave mirror produces a virtual and enlarged image.
An object is kept on the principal axis of a concave mirror of focal length 10 cm. at a distance of 15
cm from its pole. The image formed by the mirror is:
(a) Virtual and magnified
(b) Virtual and diminished
(c) Real and magnified
(d) Real and diminished
Use the mirror equation to show that an object placed between f and 2f of a concave mirror forms an image beyond 2f.
Define the term 'limit of resolution'?
Use the mirror equation to show a convex mirror always produces a virtual image independent of the location of the object ?
Mark the correct options.
A light ray falling at an angle of 45° with the surface of a clean slab of ice of thickness 1.00 m is refracted into it at an angle of 30°. Calculate the time taken by the light rays to cross the slab. Speed of light in vacuum = 3 × 108 m s−1.
A light ray is incident at an angle of 45° with the normal to a √2 cm thick plate (μ = 2.0). Find the shift in the path of the light as it emerges out from the plate.
Find the maximum angle of refraction when a light ray is refracted from glass (μ = 1.50) to air.
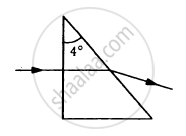
Find the angle of deviation suffered by the light ray shown in figure. The refractive index μ = 1.5 for the prism material.
A light ray, going through a prism with the angle of prism 60°, is found to deviate by 30°. What limit on the refractive index can be put from these data?
Write any one use for each of the following mirrors :
(a) Convex
(b) Concave
Name the physical principle on which the working of optical fibers is based.
For paraxial rays, show that the focal length of a spherical mirror is one-half of its radius of curvature.
A parallel beam of light is allowed to fall on a transparent spherical globe of diameter 30cm and refractive index 1.5. The distance from the centre of the globe at which the beam of light can converge is ______ mm.
An upright object is placed at a distance of 40 cm in front of a convergent lens of a focal length of 20 cm. A convergent mirror of focal length 10 cm is placed at a distance of 60 cm on the other side of the lens. The position and size of the final image will be ______.
