Advertisements
Advertisements
प्रश्न
Using Gauss's law in electrostatics, deduce an expression for electric field intensity due to a uniformly charged infinite plane sheet. If another identical sheet is placed parallel to it, show that there is no electric field in the region between the two sheets ?
Advertisements
उत्तर
Electric Field Due to a uniformly charged infinitely large plane thin sheet with surface charge density σ, using Gauss's law
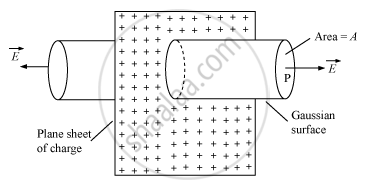
Consider an infinite thin plane sheet of positive charge with a uniform surface charge density σ on both sides of the sheet. Let P be the point at a distance a from the sheet at which the electric field is required. Draw a Gaussian cylinder of area of cross-section A through point P.
The electric flux crossing through the Gaussian surface,
Φ = E × Area of the circular caps of the cylinder
Since electric lines of force are parallel to the curved surface of the cylinder, the flux due to the electric field of the plane sheet of charge passes only through the two circular caps of the cylinder.
Φ = E × 2A … (1)
According to Gauss' Theorem,
`ø q/ε_0`
Here, the charge enclosed by the Gaussian surface,
q = σA
`ø = (σA)/c_0` ...... (2)
From equations (i) and (ii), we get:
`Exx 2A =(σA)/ε_0`
`E = σ/(2ε_0)`
Consider two infinite plane parallel sheets of charge A and B. Let σ1 and σ1 be the uniform surface charge on A and B respectively.
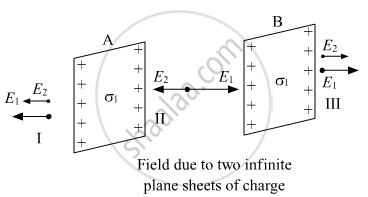
Therefore net electric field (E) between the two sheets can be found out as follows:
\[\text { Electric field due to sheet A }\]
\[ E_1 = \frac{\sigma_1}{2 \epsilon_o} \]
\[\text { Electric field due to sheet B }\]
\[ E_2 = \frac{\sigma_1}{2 \epsilon_o}\]
\[E = E_1 - E_2 \]
\[ = \frac{\sigma_1}{2 \epsilon_o} - \frac{\sigma_1}{2 \epsilon_o} = 0\]
Hence, there is no electric field in the region between the two sheets.
APPEARS IN
संबंधित प्रश्न
Use Gauss's law to find the electric field due to a uniformly charged infinite plane sheet. What is the direction of field for positive and negative charge densities?
Two large, thin metal plates are parallel and close to each other. On their inner faces, the plates have surface charge densities of opposite signs and of magnitude 17.0 × 10−22 C/m2. What is E:
- in the outer region of the first plate,
- in the outer region of the second plate, and
- between the plates?
An infinitely large thin plane sheet has a uniform surface charge density +σ. Obtain the expression for the amount of work done in bringing a point charge q from infinity to a point, distant r, in front of the charged plane sheet.
A small conducting sphere of radius 'r' carrying a charge +q is surrounded by a large concentric conducting shell of radius Ron which a charge +Q is placed. Using Gauss's law, derive the expressions for the electric field at a point 'x'
(i) between the sphere and the shell (r < x < R),
(ii) outside the spherical shell.
Using Gauss’s law, prove that the electric field at a point due to a uniformly charged infinite plane sheet is independent of the distance from it.
A spherical shell made of plastic, contains a charge Q distributed uniformly over its surface. What is the electric field inside the shell? If the shell is hammered to deshape it, without altering the charge, will the field inside be changed? What happens if the shell is made of a metal?
A thin, metallic spherical shell contains a charge Q on it. A point charge q is placed at the centre of the shell and another charge q1 is placed outside it as shown in the following figure . All the three charges are positive. The force on the charge at the centre is ____________.
A large non-conducting sheet M is given a uniform charge density. Two uncharged small metal rods A and B are placed near the sheet as shown in the following figure.
(a) M attracts A.
(b) M attracts B.
(c) A attracts B.
(d) B attracts A.
“A uniformly charged conducting spherical shell for the points outside the shell behaves as if the entire charge of the shell is concentrated at its centre”. Show this with the help of a proper diagram and verify this statement.
