Advertisements
Advertisements
प्रश्न
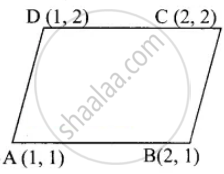
Verify that the following points taken in order to form the vertices of a rhombus
A(1, 1), B(2, 1), C(2, 2) and D(1, 2)
उत्तर
Distance = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2`
AB = `sqrt((2 - 1)^2 + (1 - 1)^2`
= `sqrt((1)^2 + (0)^2`
= `sqrt(1)`
= 1
BC = `sqrt((2 - 2)^2 + (2 - 1)^2`
= `sqrt((0)^2 + (1)^2`
= `sqrt(1)`
= 1
CD = `sqrt((1 - 2)^2 + (2 - 2)^2`
= `sqrt((- 1)^2 + (0)^2`
= `sqrt(1)`
= 1
AD = `sqrt((1 - 1)^2 + (2 - 1)^2`
= `sqrt((0)^2 + (1)^2`
= `sqrt(1)`
= 1
AB = BC = CD = AD = 1.
All the four sides are equal.
∴ ABCD is a rhombus.
APPEARS IN
संबंधित प्रश्न
If the co-ordinate of A is x and that of B is y, find d(A, B).
x = -4, y = -5
Sketch proper figure and write the answer of the following question.
If A- B - C and l(AC) = 11, l(BC) = 6.5, then l(AB) = ?
On a number line, co-ordinates of P, Q, R are 3, -5 and 6 respectively. State with reason whether the following statement is true or false.
d(P, Q) - d(P, R) = d(Q, R)
Co-ordinates of the pair of a point is given below. Hence find the distance between the pair.
3, 6
If P-Q-R and d(P, Q) = 3.4, d(Q, R)= 5.7 then d(P, R) = ?
Show that the following points taken in order to form an isosceles triangle
A(5, 4), B(2, 0), C(−2, 3)
Show that the following points taken in order to form an equilateral triangle
`"A"(2, 2), "B"(-2, -2), "C"(-2sqrt(3), 2sqrt(3))`
Show that the following points taken in order to form the vertices of a parallelogram
A(−7, −3), B(5, 10), C(15, 8) and D(3, −5)
The abscissa of a point A is equal to its ordinate, and its distance from the point B(1, 3) is 10 units, What are the coordinates of A?
Find the distance with the help of the number line given below.

d(J, H)
