Advertisements
Advertisements
प्रश्न
Write an expression for the resistance of a conducting wire in terms of its length and area of cross-section.
उत्तर
Let R be the resistance of a conducting wire of length l and area of cross-section A. We then have:
R = ρl/A
Here ρ is a constant characteristic of the material of the wire and is called its resistivity. Thus,
Resistance = `(("Resistivity of the material of the wire") xx ("length of thge wire"))/("Area of cross-section of the wire")`
APPEARS IN
संबंधित प्रश्न
In series combination which remains constant?
(a) Voltage
(b) Current
(c) Both current and voltage
(d) Both are variables
Two resistors of 4Ω and 6 Ω are connected in parallel to a cell to draw 0.5 A current from the cell.
(i) Draw a labelled circuit diagram showing the above arrangement.
(ii) Calculate the current in each resistor. What is an Ohmic resistor?
State the order of resistivity of (i) a metal, (ii) a semiconductor and (iii) an insulator.
State how are the two resistors joined with a battery when equivalent resistance is more than either of the two resistances.
You have three resistors of values 2 `Omega`, 3 `Omega`, and 5 `Omega`. How will you join them so that the total resistance is less than 2 `Omega`?
To increase the effective resistance in a circuit, the resistors are connected in _______________ .
What is a resistance? Define it with respect to Ohm’s law,
Six equal resistors of 1 ohm each are connected to form the sides of a hexagon ABCDEF. Calculate the resistance offered by the combination if the current enters at A and leaves it at D.
Two wires of the same length and area made of two materials of resistivity ρ1 and ρ2 are connected in series to a source of potential V. The equivalent resistivity for the same area is:
Consider the following combinations of resistors:
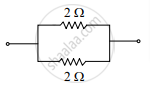



The combinations having equivalent resistance 1 Q is/are ______.
