Question

A(−3, 0) B(10, −2) and C(12, 3) are the vertices of ∆ABC. Find the equation of the altitude through A and B.

Answer 1

To find the equation of the altitude from A.

The vertices of ∆ABC are A(−3, 0) B(10, −2) and C(12, 3)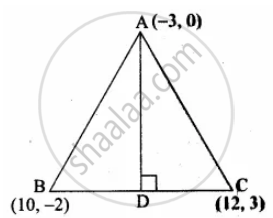

Slope of BC = `(y_2 - y_1)/(x_2 - x_1)`

= `(3 + 2)/(12 - 10)`

= `5/2`

Slope of the altitude AD is `-2/5`

Equation of the altitude AD is

y – y₁ = m (x – x₁)

y – 0 = `-2/5(x + 3)`

5y = −2x − 6

2x + 5y + 6 = 0

Equation of the altitude AD is 2x + 5y + 6 = 0

Equation of the altitude from B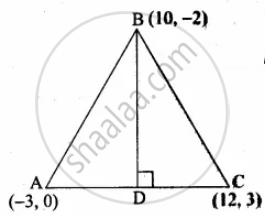

Slope of AC = `(3 - 0)/(12 + 3) = 3/15 = 1/5`

Slope of the altitude AD is − 5

Equation of the altitude BD is y – y₁= m (x – x₁)

y + 2 = – 5 (x – 10)

y + 2 = – 5x + 50

5x + y + 2 – 50 = 0

⇒ 5x + y – 48 = 0

Equation of the altitude from B is 5x + y – 48 = 0