Advertisements
Advertisements
प्रश्न
A and B can finish a work in 20 days. A alone can do \[\frac{1}{5}\] th of the work in 12 days. In how many days can B alone do it?
उत्तर
\[\text{ It is given that A and B can finish the work in 20 days }. \]
\[ \therefore \text{ Work done by } \left( A + B \right) \text{ in 1 day } = \frac{1}{20}\]
\[\text{ Now, A alone can do } \frac{1}{5}\text{ th of the work in 12 days } . \]
\[ \therefore \text { Time taken by A alone to complete the work } = \left( 5 \times 12 \right) = 60 \text{ days} \]
\[ \Rightarrow \text{ Work done by A in 1 day } = \frac{1}{60}\]
\[\text{ Now, work done by B in 1 day = Work done by } \left( A + B \right) \text{ in 1 day work - Work done by A in 1 day } \]
\[ = \frac{1}{20} - \frac{1}{60}\]
\[ = \frac{3 - 1}{60} = \frac{2}{60}\]
\[\text{ Thus, B alone can polish the floor in } \frac{60}{2}\text{ days or 30 days } . \]
APPEARS IN
संबंधित प्रश्न
A 5 m 60 cm high vertical pole casts a shadow 3 m 20 cm long. Find at the same time the length of the shadow cast by another pole 10 m 50 cm high.
Aasheesh can paint his doll in 20 minutes and his sister Chinki can do so in 25 minutes. They paint the doll together for five minutes. At this juncture they have a quarrel and Chinki withdraws from painting. In how many minutes will Aasheesh finish the painting of the remaining doll?
Two taps A and B can fill an overhead tank in 10 hours and 15 hours respectively. Both the taps are opened for 4 hours and they B is turned off. How much time will A take to fill the remaining tank?
A cistern has two inlets A and B which can fill it in 12 hours and 15 hours respectively. An outlet can empty the full cistern in 10 hours. If all the three pipes are opened together in the empty cistern, how much time will they take to fill the cistern completely?
If x varies directly as y, then
| x | 12 | 6 |
| y | 48 | – |
If the thickness of a pile of 12 cardboard sheets is 45 mm, then the thickness of a pile of 240 sheets is ______ cm.
Length of a side of a square and its area vary directly with each other.
Campus and Welfare Committee of school is planning to develop a blue shade for painting the entire school building. For this purpose various shades are tried by mixing containers of blue paint and white paint. In the following mixtures, decide which is a lighter shade of blue and also find the lightest blue shade among all of them.
| Mixture G | Mixture H |
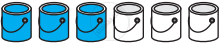 |
 |
If one container has one litre paint and the building requires 105 litres for painting, how many container of each type is required to paint the building by darkest blue shade?
30 persons can reap a field in 17 days. How many more persons should be engaged to reap the same field in 10 days?
Match each of the entries in Column I with the appropriate entry in Column II
| Column I | Column II |
| 1. x and y vary inversely to each other | A. `x/y` = Constant |
| 2. Mathematical representation of inverse variation of quantities p and q |
B. y will increase in proportion |
| 3. Mathematical representation of direct variation of quantities m and n |
C. xy = Constant |
| 4. When x = 5, y = 2.5 and when y = 5, x = 10 | D. `p oo 1/q` |
| 5. When x = 10, y = 5 and when x = 20, y = 2.5 | E. y will decrease in proportion |
| 6. x and y vary directly with each other | F. x and y are directly proportional |
| 7. If x and y vary inversely then on decreasing x | G. `m oo n` |
| 8. If x and y vary directly then on decreasing x | H. x and y vary inversely |
| I. `p oo q` | |
| J. `m oo 1/n` |
