Advertisements
Advertisements
प्रश्न
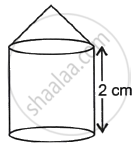
A right circular cylinder just encloses a sphere of radius r units. Calculate the curved surface area of the cylinder
उत्तर
C.S.A of cylinder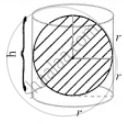
= 2πr × h
= 2πr × 2r
= 4πr2 sq.units
APPEARS IN
संबंधित प्रश्न
A farmer connects a pipe of internal diameter 20 cm from a canal into a cylindrical tank which is 10 m in diameter and 2 m deep. If the water flows through the pipe at the rate of 4 km per hour, in how much time will the tank be filled completely?
The dimensions of a cuboid are 44 cm, 21 cm, 12 cm. It is melted and a cone of height 24 cm is made. Find the radius of its base.
In the given figure, a cylindrical wrapper of flat tablets is shown. The radius of a tablet is 7 mm and its thickness is 5 mm. How many such tablets are wrapped in the wrapper?

In the given figure shows a toy. Its lower part is a hemisphere and the upper part is a cone. Find the volume and surface area of the toy from the measures shown in the figure (\[\pi = 3 . 14\])
A tin maker converts a cubical metallic box into 10 cylindrical tins. The side of the cube is 50 cm and the radius of the cylinder is 7 cm. Find the height of each cylinder so made, if the wastage of 12% is incurred in the process `(pi = 22/7)`
A vessel is in the form of a hemispherical bowl mounted by a hollow cylinder. The diameter is 14 cm and the height of the vessel is 13 cm. Find the capacity of the vessel
A solid consisting of a right circular cone of height 12 cm and radius 6 cm standing on a hemisphere of radius 6 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of the water displaced out of the cylinder, if the radius of the cylinder is 6 cm and height is 18 cm
A right circular cylinder just encloses a sphere of radius r units. Calculate the surface area of the sphere
Find volume of solid generated by revolving parabola y2. = 4ax, cut of by latus rectum, about tangent at vertex.
A cylinder and a cone have equal bases. The height of the cylinder is 2 cm and the area of its base is 64 cm2. The cone is placed upon the cylinder volume of the solid figure so formed is 400 cm3. Find the total height of the figure.