Advertisements
Advertisements
प्रश्न
Altitudes MN and MO of parallelogram MGHK are 8 cm and 4 cm long respectively in the below figure. One side GH is 6 cm long. Find the perimeter of MGHK.

उत्तर
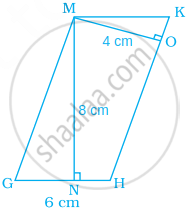
Given, MGHK is a parallelogram, where MN = 8 cm, MO = 4 cm and GH = 6 cm
∴ Area of parallelogram MGHK, when base is GH ......[∵ Area of parallelogram = Base × Height]
= GH × MN
= 6 × 8 cm2
= 48 cm2 ......(i)
Area of paralielogram MGHK, when base is HK
= HK × MO
⇒ 48 = KH × 4 ......[From equation (i)]
⇒ HK = `48/4`
⇒ HK = 12 cm
In parallelogram, opposite sides are equal
So, GH = MK = 6 cm and MG = HK = 12 cm
∴ Perimeter of paralielogram MGHK = (6 + 6 + 12 + 12) cm = 36 cm.
APPEARS IN
संबंधित प्रश्न
A parallelogram has sides of 15 cm and 12 cm; if the distance between the 15 cm sides is 6 cm; find the distance between 12 cm sides.
Find the missing values.
| Base | Height | Area |
| 8 cm | 56 sq.m |
Find the missing values.
| Base | Height | Area |
| 17 mm | 221 sq.mm |
The base of the parallelogram with area is 52 sq.cm and height 4 cm is
In a parallelogram the base is three times its height. If the height is 8 cm then the area is
If the base and height of a parallelogram are in the ratio 7 : 3 and the height is 45 cm, then fixed the area of the parallelogram
Find the height of the parallelogram whose base is four times the height and whose area is 576 sq.cm
Observe the shapes 1, 2, 3 and 4 in the figures. Which of the following statements is not correct?
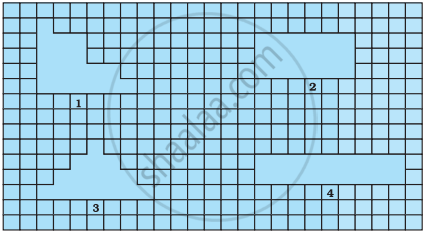
All parallelograms having equal areas have same perimeters.
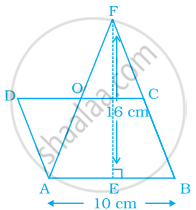
In the given figure, area of ∆AFB is equal to the area of parallelogram ABCD. If altitude EF is 16 cm long, find the altitude of the parallelogram to the base AB of length 10 cm. What is the area of ∆DAO, where O is the midpoint of DC?