Advertisements
Advertisements
प्रश्न
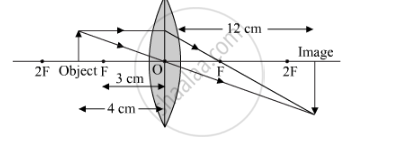
An object placed 4 cm in front of a converging lens produces a real image 12 cm from the lens.
(a) What is the magnification of the image?
(b) What is the focal length of the lens?
(c) Draw a ray diagram to show the formation of image. Mark clearly F and 2F in the diagram.
उत्तर
Converging lens is a convex lens.
Distance of the object from the lens (u) =-4
Distance of the image from the lens (v) = 12
(a) Magnification of the lens is given by:
`m=v/u`
⇒`m=12/-4=-3`
Thus , magnifiation produced by the llens 3.
(b) Lens formula is given by:
`1/f=1/v-1/u`
⇒`1/f=1/12-1/-4`
⇒`1/f=1/12+1/4`
⇒`1/f=(1+3)/12`
⇒`f=3`cm
(c)
APPEARS IN
संबंधित प्रश्न
Where should an object be placed in front of the concave mirror so as to obtain its virtual, erect and magnified image?
Define linear magnification produced by a mirror.
Fill in the following blank with suitable word:
If the magnification has a minus sign, than the image is ......... and .......
Write down the magnification formula for a lens in terms of object distance and image distance. How does this magnification formula for a lens differ from the corresponding formula for a mirror?
An object is placed at a distance of 100 cm from a converging lens of focal length 40 cm.
(i) What is the nature of image?
(ii) What is the position of image?
Determine how far an object must be placed in front of a converging lens of focal length 10 cm in order to produce an erect (upright) image of linear magnification 4.
The magnification produced by a spherical lens and a spherical mirror is + 2.0.
(a) The lens and mirror are both concave
(b) The lens and mirror are both convex
(c) The lens is convex but the mirror is concave
(d) The lens is concave but the mirror is convex
Magnification produced by a rear view mirror fitted in vehicles ______.
Assertion: The focal length of the mirror is /and the distance of the object from the focus is V then the magnification of the mirror will be `("f"/"f−u")`
Reason: Magnification = `"image distance"/ "object distance"` = `(-"v"/"u")`
What do you mean by linear magnification?
