Advertisements
Advertisements
प्रश्न
Answer the following question.
Describe any two characteristic features which distinguish between interference and diffraction phenomena. Derive the expression for the intensity at a point of the interference pattern in Young's double-slit experiment.
Advertisements
उत्तर
Difference between interference and diffraction:
In the interference pattern, the intensity of the dark fringe is completely zero.
In the diffraction pattern, the intensity of secondary minima is minimum, but not completely zero.
In interference pattern the width of all the interference fringes is equal. In diffraction pattern the width of central maxima is large, and on increasing distance, the width of maxima decreases.
In interference pattern the intensity of all the bright bands is equal.
In the diffraction pattern, the intensity of all the secondary maxima is not equal.
The principle of superposition of light waves:
When two or more wave trains of light travelling in a medium superpose upon each other, the resultant displacement at any instant is equal to the vector sum of the displacements due to individual waves.
If `vecy_1,vecy_2,vecy_3,....` be the displacements due to different waves, then the resultant displacement is given by, `vecy = vecy_1 + vecy_2 + vecy_3 +` ... conditions for constructive and destructive interference:
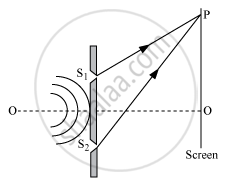
Let the displacement of the waves from the source `S_1` and `S_2` at a point P on the screen at any time 't' be given by,
`"y"_1 = a_1 sin omegat`
and
`"y"_2 = a_2 sin(omegat + phi)`
where `phi` is the constant phase difference between the two waves
by the superposition principal , the resultant displacement at point P is given by,
`"y" = "y"_1 + "y"_2`
= `a_1 sin omegat + a_2 sin (omegat + phi)`
= `a_1 sin omegat + a_2 sin omegat cos phi + a_2 cos omegat sin phi`
`y = (a_1 + a_2 cos phi) sin omegat + a_2 sin phi cos omegat` ...(i)
Let `a_1 + a_2 cos phi = A cos theta` ...(ii)
`a_2 sin phi = A sin theta` ...(iii)
Then, equation (i) becomes
`"y" = Acostheta sin omegat + A sin theta cos omegat`
`"y" = Asin(omegat + theta)`
Squaring and adding both sides of the equations (ii) and (iii), we obtain
`A^2 cos^2theta + A^2 sin^2 theta = (a_1 + a_2 cos phi)^2 + a_2^2 sin^2 phi`
`A^2 = a_1^2 + a_2^2 (cos^2phi + sin^2phi) + 2a_1a_2 cos phi`
`A^2 = a_1^2 + a_2^2 + 2a_1a_2 cos phi`
The intensity of light is directly proportional to the square of the amplitude of the wave. The intensity of light at point P on the screen is given by,
`I = a_1^2 + a_2^2 + 2a_1a_2 cos phi` ...(iv)
संबंधित प्रश्न
Four light waves are represented by
(i) \[y = a_1 \sin \omega t\]
(ii) \[y = a_2 \sin \left( \omega t + \epsilon \right)\]
(iii) \[y = a_1 \sin 2\omega t\]
(iv) \[y = a_2 \sin 2\left( \omega t + \epsilon \right).\]
Interference fringes may be observed due to superposition of
(a) (i) and (ii)
(b) (i) and (iii)
(c) (ii) and (iv)
(d) (iii) and (iv)
A long narrow horizontal slit is paced 1 mm above a horizontal plane mirror. The interference between the light coming directly from the slit and that after reflection is seen on a screen 1.0 m away from the slit. If the mirror reflects only 64% of the light energy falling on it, what will be the ratio of the maximum to the minimum intensity in the interference pattern observed on the screen?
Answer in brief:
In Young's double-slit experiment what will we observe on the screen when white light is incident on the slits but one slit is covered with a red filter and the other with a violet filter? Give reasons for your answer.
A double-slit arrangement produces interference fringes for sodium light (λ = 589 nm) that are 0.20° apart. What is the angular fringe separation if the entire arrangement is immersed in water (n = 1.33)?
One of Young’s double slits is covered with a glass plate as shown in figure. The position of central maximum will,
A thin mica sheet of thickness 4 x 10-6 m and refractive index 1.5 is introduced in the path of the first wave. The wavelength of the wave used is 5000 A. The central bright maximum will shift ______.
In Young's double slit experiment, for wavelength λ1 the nth bright fringe is obtained at a point P on the screen. Keeping the same setting, source of light is replaced by wavelength λ2 and now (n + 1)th bright fringe is obtained at the same point P on the screen. The value of n is ______.
A double slit experiment is immersed in water of refractive index 1.33. The slit separation is 1 mm, distance between slit and screen is 1.33 m. The slits are illuminated by a light of wavelength 6300 Å. The fringe width is ______.
In Young's double-slit experiment, the distance between the slits is 3 mm and the slits are 2 m away from the screen. Two interference patterns can be obtained on the screen due to light of wavelength 480 nm and 600 run respectively. The separation on the screen between the 5th order bright fringes on the two interference patterns is ______
How will the interference pattern of Young's double slit change if one of the two slits is covered by a paper which transmits only half of the light intensity?
