Advertisements
Advertisements
प्रश्न
As shown in figure, LK = `6sqrt(2)` then

- MK = ?
- ML = ?
- MN = ?
उत्तर
(i) In ∆MLK,
∠MLK = 90° and ∠LKM = 30° ......[Given]
∴ ∠LMK = 60° ......[Remaining angle of a triangle]
∴ ∆MLK is a 30° – 60° – 90° triangle.
∴ LK = `sqrt(3)/2` MK ......[Side opposite to 60°]
∴ `6sqrt(2) = sqrt(3)/2` MK ......[Given]
∴ MK = `6sqrt(2) xx 2/sqrt(3)`
= `(12sqrt(2))/sqrt(3)`
= `(12 xx sqrt(2) xx sqrt(3))/(sqrt(3) xx sqrt(3))` ......[Multiply numerator and denominator by `sqrt(3)`]
= `(12 xx sqrt(2 xx 3))/3`
MK = `4sqrt(6)` units
(ii) In ∆MLK,
∠MLK = 90° ......[Given]
∴ MK2 = ML2 + LK2 ......[Pythagoras theorem]
∴ `(4sqrt(6))^2 = "ML"^2 + (6sqrt(2))^2` ......[From (i) and given]
∴ (16 × 6) = ML2 + (36 × 2)
∴ 96 = ML2 + 72
∴ ML2 = 24
∴ ML = `sqrt(24)` .......[Taking square root of both sides]
∴ ML = `sqrt(4 xx 6)`
∴ ML = `2sqrt(6)` units
(iii) In ∆NKM,
∠NKM = 90° and ∠MNK = 45° ......[Given]
∴ ∠KMN = 45° ......[Remaining angle of a triangle]
∴ ∆NKM is 45° – 45° – 90° triangle.
∴ MK = `1/sqrt(2)` MN ......[Theorem of 45° – 45° – 90° triangle]
∴ `4sqrt(6) = 1/sqrt(2)` MN ......[From (i)]
∴ MN = `4sqrt(6) xx sqrt(2)`
= `4sqrt(6 xx 2)`
= `4sqrt(4 xx 3)`
= `4 xx2 xx sqrt(3)`
∴ MN = `8sqrt(3)` units
संबंधित प्रश्न
In the following figure, in ΔABC, ∠B = 90°, ∠C = 60°, ∠A = 30°, AC = 18 cm. Find BC.

Find the height of an equilateral triangle whose side is 6 units.
In the given figure. Find RP and PS using the information given in ∆PSR.

In the given figure, ∆PQR is an equilateral triangle. Point S is on seg QR such that QS = n\[\frac{1}{3}\] QR.
Prove that: 9 PS2 = 7 PQ2
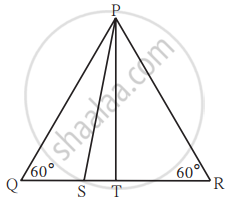
In right-angled triangle PQR, if ∠P = 60°, ∠R = 30° and PR = 12, then find the values of PQ and QR.
In ΔDEF, if ∠E = 90°, then find the value of ∠D + ∠F.
Choose the correct alternative:
Out of given triplets, which is a Pythagoras triplet?
Choose the correct alternative:
The diagonal of a square is `10sqrt(2)` cm then its perimeter is ______
From given figure, In ∆ABC, If ∠ABC = 90° ∠CAB=30°, AC = 14 then for finding value of AB and BC, complete the following activity.
Activity: In ∆ABC, If ∠ABC = 90°, ∠CAB=30°
∴ ∠BCA = `square`
By theorem of 30° – 60° – 90° triangle,
∴ `square = 1/2` AC and `square = sqrt(3)/2` AC
∴ BC = `1/2 xx square` and AB = `sqrt(3)/2 xx 14`
∴ BC = 7 and AB = `7sqrt(3)`
From given figure, in ∆MNK, if ∠MNK = 90°, ∠M = 45°, MK = 6, then for finding value of MN and KN, complete the following activity.

Activity:
In ∆MNK, ∠MNK = 90°, ∠M = 45° …...[Given]
∴ ∠K = `square` .....[Remaining angle of ∆MNK]
By theorem of 45° – 45° – 90° triangle,
∴ `square = 1/sqrt(2)` MK and `square = 1/sqrt(2)` MK
∴ MN = `1/sqrt(2) xx square` and KN = `1/sqrt(2) xx 6`
∴ MN = `3sqrt(2)` and KN = `3sqrt(2)`
In ∆RST, ∠S = 90°, ∠T = 30°, RT = 12 cm, then find RS.
