Advertisements
Advertisements
प्रश्न
By the graphical method, find whether the following pair of equations are consistent or not. If consistent, solve them.
x + y = 3, 3x + 3y = 9
उत्तर
Given pair of equation are
x + y = 3
⇒ x + y – 3 = 0 ......(i)
And 3x + 3y = 9
⇒ 3x + 3y – 9 = 0 .......(ii)
On comparing with ax + by + c = 0, we get
a1 = 1, b1 = 1 and c1 = –3 ......[From (i)]
a2 = 3, b2 = 3 and c2 = –9 ......[From (ii)]
Here, `a_1/a_2 = 1/3`,
`b_1/b_2 = 1/3`
And `c_1/c_2 = (-3)/(-9) = 1/3`
⇒ `a_1/a_2 = b_1/b_2 = c_1/c_2`
So, the given pair of lines is coincident.
Therefore, these lines have infinitely many solutions.
Hence, the given pair of linear equations are consistent.
Now, x + y = 3
⇒ y = 3 – x
| x | 0 | 3 | 2 |
| y | 3 | 0 | 1 |
And 3x + 3y = 9
⇒ 3y = 9 – 3x
⇒ y = `(9 - 3x)/3`
| x | 0 | 1 | 3 |
| y | 3 | 2 | 0 |
Plotting the points we get the graph of lines.
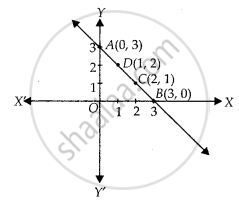
We observe that the lines represented by (i) and (ii) are coincident.
