Advertisements
Advertisements
प्रश्न
Can a triangle have two obtuse angles? Justify your answer in case.
उत्तर
No,
A triangle can’t have 2 obtuse angles. Obtuse angle means more than `90^@` So that the
sum of the two sides will exceed `180^@`which is not possible. As the sum of all three
angles of a triangle is `180^@` .
APPEARS IN
संबंधित प्रश्न
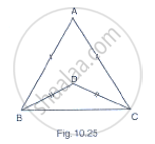
In Fig. 10.25, AB = AC and DB = DC, find the ratio ∠ABD : ∠ACD.
In ΔABC, ∠B = ∠C and ray AX bisects the exterior angle ∠DAC. If ∠DAX = 70°, then ∠ACB =
An exterior angle of a triangle is 108° and its interior opposite angles are in the ratio 4 : 5. The angles of the triangle are
In the following, find the marked unknown angle:

Can a triangle together have the following angles?
33°, 74° and 73°
Find, giving a reason, the unknown marked angles, in a triangle drawn below:
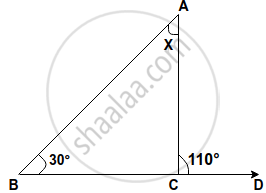
Find, giving a reason, the unknown marked angles, in a triangle drawn below:

The length of the sides of the triangle is given. Say what types of triangles they are 4.3 cm, 4.3 cm, 4.3 cm.
The length of the sides of the triangle is given. Say what types of triangles they are 3.7 cm, 3.4 cm, 4 cm.
Can 30°, 60° and 90° be the angles of a triangle?
