Advertisements
Advertisements
प्रश्न
Draw a circle of radius 4.2 cm. Draw arc PQ measuring 120°. Draw a tangent to the circle from point P and point Q
उत्तर
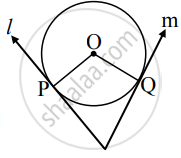
Analysis:
Let O be the centre of the circle.
Here, ∠POQ = m(arc PQ) ......[Definition of measure of minor arc]
∴ On drawing ∠POQ = 120°, we get an arc
PQ measuring 120°.
line l and line m are tangents to the circle.
line l ⊥ seg OP and line m ⊥ seg OQ ......[Tangent theorem]
∴ To get tangents l and m, we draw perpendiculars to seg OP and seg OQ at points P and Q respectively.
Steps of construction:
- Draw a circle of radius 4.2 cm with centre O.
- Draw rays OP and OQ such that ∠POQ = 120°. (Points P and Q must be on the circle.)
- Draw line l ⊥ ray OP at point P
- Draw line m ⊥ ray OQ at point Q.
Line l and m are the required tangents.
APPEARS IN
संबंधित प्रश्न
Construct a tangent to a circle with centre P and radius 3.2 cm at any point M on it.
Draw a circle of radius 2.7 cm. Draw a tangent to the circle at any point on it.
Draw any circle. Take any point A on it and construct tangent at A without using the centre of the circle.
Draw a circle with centre P. Draw an arc AB of 100° measure. Draw tangents to the circle at point A and point B.
Construct tangent to a circle with centre A and radius 3.4 cm at any point P on it.
Draw a circle of radius 3 cm and draw a tangent to the circle from point P on the circle
| Draw a circle with center O and radius 3 cm |
| ↓ |
| Take any point P on the circle |
| ↓ |
| Draw ray OP |
| ↓ |
| Draw perpendicular to ray OP from point P |
Draw a circle of radius 4.2 cm, take any point M on it. Draw tangent to the circle from point M
Do the following activity to draw tangents to the circle without using the center of the circle.
- Draw a circle with radius 3.5 cm and take any point C on it.
- Draw chord CB and an inscribed angle CAB.
- With the center A and any convenient radius, draw an arc intersecting the sides of angle BAC in points M and N.
- Using the same radius, draw an arc intersecting the chord CB at point R.
- Taking the radius equal to d(MN) and center R, draw an arc intersecting the arc drawn in the previous step. Let D be the point of intersection of these arcs. Draw line CD. Line CD is the required tangent to the circle.
Draw a circle with center O and radius 3.4. Draw a chord MN of length 5.7 cm in a circle. Draw tangents to the circle from point M and N
Draw a circle with center O and radius 3.6 cm. Draw a tangent to the circle from point B at a distance of 7.2 cm from the center of the circle.
Draw a circle with center C and radius 3.2 cm. Draw a tangent to the circle from point P at a distance of 7.5 cm from the center of the circle
Draw a circle with a radius of 3.5 cm. Take the point K anywhere on the circle. Draw a tangent to the circle from K (without using the center of the circle)
Draw a circle with radius 3 cm. Construct a square such that each of its side will touch the circle from outside
Take point P and Q and draw a circle passing through them. Draw a tangent AB to the circle without using the centre of the circle.
Draw any circle with radius greater than 1.8 cm and less than 3 cm. Draw a chord AB 3.6 cm long in this circle. Tangent to the circle passing through A and B without using the center of the circle
Draw a circle with center O and radius 2.8 cm. Take point P in the exterior of a circle such that tangents PA and PB drawn from point P make an angle ∠APB of measure 70°
Draw a circle of radius 3.2 cm and centre 'O'. Take any point P on it. Draw tangent to the circle through point P using the centre of the circle.
Draw a circle of suitable radius. Take point T on it. Draw a tangent through point T.
