Advertisements
Advertisements
प्रश्न
Draw five triangles and measure their sides. Check-in each case, if the sum of the lengths of any two sides is always less than the third side.
उत्तर
Case 1. Let us draw a triangle ABC
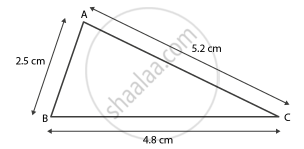
AB = 2.5 cm
BC = 4.8 cm and
AC = 5.2 cm
AB + BC = 2.5 cm + 4.8 cm
= 7.3 cm
As 7.3 > 5.2
∴ AB + BC > AC
Thus, we observe that the sum of any two sides of a triangle is greater than the third side.
Case 2. Let us draw a triangle PQR
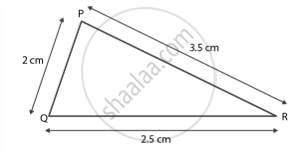
PQ = 2 cm
QR = 2.5 cm
PR = 3.5 cm
PQ + QR = 2 cm + 2.5 cm
= 4.5 cm
As 4.5 > 3.5
∴ PQ + QR > PR
Thus, we observe that the sum of any two sides of a triangle is greater than the third side.
Case 3. Let us draw triangle XYZ
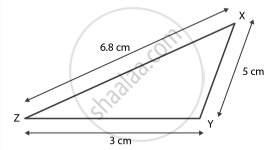
XY = 5 cm
YZ = 3 cm
ZX = 6.8 cm
XY + YZ = 5 cm + 3 cm
= 8 cm
As 8 > 6.8
∴ XY + YZ > ZX
Thus, we observe that the sum of any two sides of a triangle is greater than the third side.
Case 4. Let us draw a triangle MNS
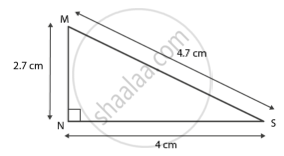
MN = 2.7 cm
NS = 4 cm
MS = 4.7 cm
MN + NS = 2.7 cm + 4 cm
6.7 cm
As 6.7 > 4.7
∴ MN + NS > MS
Thus, we observe that the sum of any two sides of a triangle is greater than the third side.
Case 5. Let us draw a triangle KLM
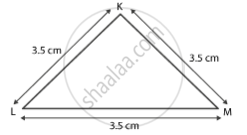
KL = 3.5 cm
LM = 3.5 cm
KM = 3.5 cm
KL + LM = 3.5 cm + 3.5 cm
= 7 cm
As 7 cm > 3.5 cm
∴ KL + LM > KM
Thus, we observe that the sum of any two sides of a triangle is greater than the third side.
Therefore we conclude that the sum of any two sides of a triangle is always greater than the third side.
APPEARS IN
संबंधित प्रश्न
Construct traingle ABC, when : AB = BC = CA = 4.5 cm. Measure all the angles of the triangle. Are they equal?
Construct traingle ABC, when : AB = 5.4 cm, ∠A = 30° and ∠B = 90°. Measure ∠C and side BC.
The angles of a triangle are (2x – 30°),(3x – 40°) and ( x + 10°) Find the value of x .
Observe the figure below and write the type of the triangle based on its angles.

Δ LMN is ... triangle
Name the type of following triangle :
∆ABC with AB = 8.7 cm, AC = 7 cm and BC = 6 cm.
Name the type of following triangle :
ΔXYZ with m∠Y = 90° and XY = YZ.
Name the following triangle in two different ways: (you may judge the nature of the angle by observation)

Name the following triangle in two different ways: (you may judge the nature of the angle by observation)

Try to construct triangles using match sticks. Some are shown here.
 |
 |
 |
Can you make a triangle with 3 matchsticks?
(Remember you have to use all the available matchsticks in each case) Name the type of triangle in each case. If you cannot make a triangle, think of reasons for it.
Draw a rough sketch of a regular octagon. (Use squared paper if you wish). Draw a rectangle by joining exactly four of the vertices of the octagon.
