Advertisements
Advertisements
प्रश्न
Draw the two tangents from a point which is 10 cm away from the centre of a circle of radius 5 cm. Also, measure the lengths of the tangents.
उत्तर
Radius = 5 cm, Distance = 10 cm


Steps of construction:
1. With O as centre, draw a circle of radius 5 cm.
2. Draw a line OP = 10 cm.
3. Draw a perpendicular bisector of OP, which cuts OP at M.
4. With M as centre and MO as radius draw a circle which cuts the previous circle at A and B.
5. Join AP and BP. AP and BP are the required tangents.
Verification: In the right ∆OAP
PA2 = OP2 – OA2
= 102 – 52
= `sqrt(100 - 25)`
= `sqrt(75)`
= 8.7 cm
Length of the tangent is = 8.7 cm
APPEARS IN
संबंधित प्रश्न
The length of the tangent to a circle from a point P, which is 25 cm away from the centre is 24 cm. What is the radius of the circle?
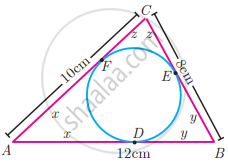
A circle is inscribed in ∆ABC having sides 8 cm, 10 cm and 12 cm as shown in the figure, Find AD, BE and CF.
A tangent ST to a circle touches it at B. AB is a chord such that ∠ABT = 65°. Find ∠AOB, where “O” is the centre of the circle.
Two circles with centres O and O’ of radii 3 cm and 4 cm, respectively intersect at two points P and Q, such that OP and O’P are tangents to the two circles. Find the length of the common chord PQ.
Draw a tangent at any point R on the circle of radius 3.4 cm and centre at P?
Draw a circle of radius 4.5 cm. Take a point on the circle. Draw the tangent at that point using the alternate segment theorem
How many tangents can be drawn to the circle from an exterior point?
The two tangents from an external points P to a circle with centre at O are PA and PB. If ∠APB = 70° then the value of ∠AOB is
CP and CQ are tangents to a circle with centre at 0. ARB is another tangent touching the circle at R. If CP = 11 cm and BC = 7 cm, then the length of BR is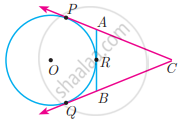
If PR is tangent to the circle at P and O is the centre of the circle, then ∠POQ is