Advertisements
Advertisements
प्रश्न
Find the values.
y = x + 3
| x | 0 | − 2 | ||
| y | 0 | − 3 |
उत्तर
Let y = x + 3
(i) if x = 0, y = 0 + 3 = 3,
∴ y = 3
(ii) y = 0, 0 = x + 3,
∴ x = – 3
(iii) x = – 2, y = – 2 + 3,
∴ y = 1
(iv) y = – 3, – 3 = x + 3,
∴ x = – 6
y = x + 3
| x | 0 | − 3 | − 2 | − 6 |
| y | 3 | 0 | 1 | − 3 |
APPEARS IN
संबंधित प्रश्न
If the point (3, 4) lies on the graph of the equation 3y = ax + 7, find the value of a.
Draw the graph of the following linear equation in two variable : –x + y = 6
Plot the points (3, 5) and (− 1, 3) on a graph paper and verify that the straight line passing
through these points also passes through the point (1, 4).
Draw the graph for the equation, given below :
y + 7 = 0
Solve, graphically, the following pairs of equation :
3x + 7y = 27
8 - y = `(5)/(2)x`
A straight line passes through the points (2, 5) and (-4, -7). Plot these points on a graph paper and draw the straight line passes through these points. If points (a, -1) and (-5, b) lie on the line drawn, find the value of a and b.
The graph of the linear equation y = x passes through the point ______.
The graph given below represents the linear equation x + y = 0.
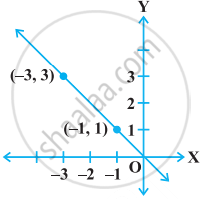
Write the linear equation such that each point on its graph has an ordinate 3 times its abscissa.
The following observed values of x and y are thought to satisfy a linear equation. Write the linear equation:
| x | 6 | – 6 |
| y | –2 | 6 |
Draw the graph using the values of x, y as given in the above table. At what points the graph of the linear equation cuts the y-axis
