Advertisements
Advertisements
प्रश्न
Given ∠POR = 3x and ∠QOR = 2x + 10, find the value of x for which POQ will be a line.
(Below fig).
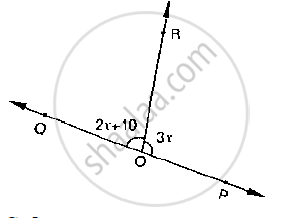
उत्तर
Since `∠`QOR, `∠`POP are linear pairs
`∠`QOR + `∠`POR = 180°
2x +10 + 3x = 180° [ ∴ `∠`QOR = 2x +10, `∠`POR = 3x]
⇒ 5x +10 = 180°
⇒ 5x = 180° -10
⇒ 5x = 170°
⇒ x = 34°
APPEARS IN
संबंधित प्रश्न
Write the supplement of the following angles .
54°
An angle is equal to 8 times its complement. Determine its measure.
In the given below Fig, find x. Further find ∠BOC, ∠COD and ∠AOD .
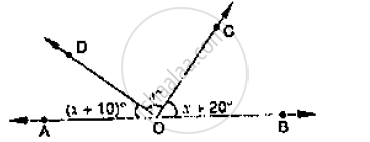
AB, CD and EF are three concurrent lines passing through the point O such that OF bisects
∠BOD. If ∠BOF = 35°, find ∠BOC and ∠AOD.
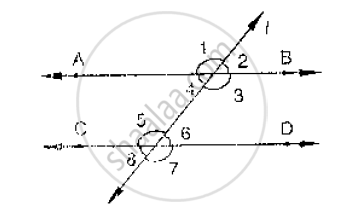
In below fig, AB CD and ∠1 and ∠2 are in the ratio 3 : 2. Determine all angles from 1 to 8.
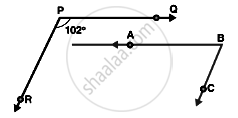
In the below, PQ || AB and PR || BC. If `∠`QPR = 102°, determine `∠`ABC. Give reasons.
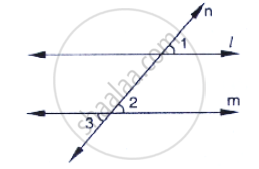
In the below fig, `∠`1 = 60° and `∠`2 = `(2/3)^(rd)`of a right angle. Prove that l || m.
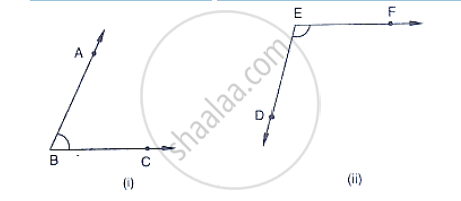
In the below fig, arms BA and BC of ∠ABC are respectively parallel to arms ED and EF of
∠DEF. Prove that ∠ABC + ∠DEF = 180°.
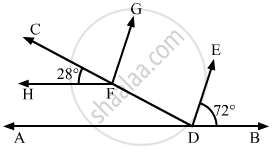
In the given figure, if AB || HF and DE || FG, then the measure of ∠FDE is
If two lines intersect, prove that the vertically opposite angles are equal.
