Advertisements
Advertisements
प्रश्न
If the bisectors of two adjacent angles A and B of a quadrilateral ABCD intersect at a point O such that ∠C + ∠D = k ∠AOB, then find the value of k.
उत्तर
The quadrilateral can be drawn as follows:

We have AO and BO as the bisectors of angles ∠A and ∠B respectively.
In ,ΔAOB We have,
∠AOB + ∠1+ ∠2 = 180°
∠AOB = 180°-(∠1 + ∠2)
∠AOB = 180° -`(1/2∠A +1/2∠B)`
`∠AOB = 180°- 1/2 (∠A+ ∠B)` …… (I)
By angle sum property of a quadrilateral, we have:
∠A+ ∠B + ∠C + ∠D = 360°
∠A+∠B = 360°-( ∠C+ ∠D)
Putting in equation (I):
`∠AOB = 180°- 1/2[360° - ( ∠C+ ∠D)]`
`∠AOB= 180° - 180° +(∠C+ ∠D)/2`
`∠AOB = 1/2 (∠C + ∠D)`
(∠C + ∠D) =2∠AOB …… (II)
On comparing equation (II) with
(∠C + ∠D) = k ∠AOB
We get k = 2.
Hence, the value for k is 2.
APPEARS IN
संबंधित प्रश्न
Define the following term Convex Quadrilateral .
A quadrilateral has all its four angles of the same measure. What is the measure of each?
In the given figure : ∠b = 2a + 15 and ∠c = 3a + 5; find the values of b and c.

In quadrilateral PQRS, ∠P : ∠Q : ∠R : ∠S = 3 : 4 : 6 : 7.
Calculate each angle of the quadrilateral and then prove that PQ and SR are parallel to each other
(i) Is PS also parallel to QR?
(ii) Assign a special name to quadrilateral PQRS.
In a trapezium ABCD, side AB is parallel to side DC. If ∠A = 78° and ∠C = 120. find angles B and D.
If three angles of a quadrilateral are 90° each, show that the given quadrilateral is a rectangle.
A quadrilateral can be drawn when all the four angles and one side is given.
In given figure, What is AC – EC?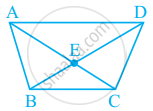
Using the information given, name the right angles in part of figure:
AE ⊥ CE
Draw a rough sketch of a quadrilateral KLMN. State two pairs of opposite sides.
