Advertisements
Advertisements
प्रश्न
The HCF of 95 and 152, is
पर्याय
57
1
19
38
उत्तर
Using the factor tree for 95, we have:
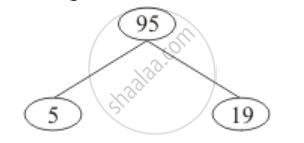
Using the factor tree for 152, we have:
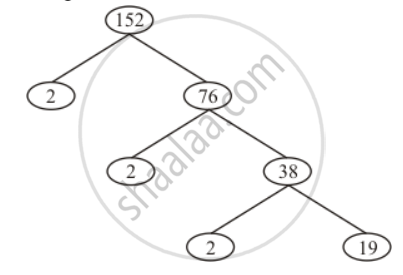
Therefore,
`95 = 5xx19`
`152=2^3xx19`
HCF (95,152)=19
Hence the correct choice is (c).
APPEARS IN
संबंधित प्रश्न
Show that every positive integer is of the form 2q and that every positive odd integer is of the from 2q + 1, where q is some integer.
Using Euclid’s algorithm, find the HCF of 405 and 2520 .
Find the greatest possible length which can be used to measure exactly the lengths 7m, 3m 85cm and 12m 95cm
The product of two numbers is 1050 and their HCF is 25. Find their LCM.
Is it possible to have two numbers whose HCF if 25 and LCM is 520?
Express each of the following integers as a product of its prime factors:
420
Express each of the following integers as a product of its prime factors:
468
Show that \[3 + \sqrt{2}\] is an irrational number.
The exponent of 2 in the prime factorisation of 144, is
A positive integer is of the form 3q + 1, q being a natural number. Can you write its square in any form other than 3m + 1, i.e., 3m or 3m + 2 for some integer m? Justify your answer.
